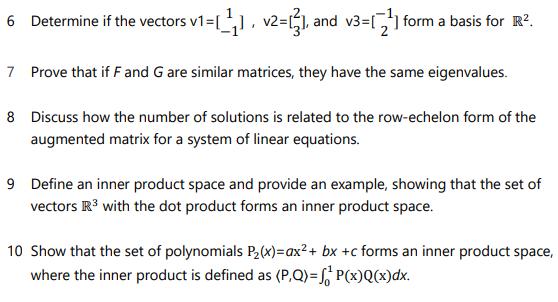
6 Determine if the vectors v1 = (1], v2=13], and v3=[1] form a basis for IR. 7 Prove that if F and G are similar matrices, they have the same eigenvalues. 8 Discuss how the number of solutions is related to the row-echelon form of the augmented matrix for a system of linear equations. 9 Define an inner product space and provide an example, showing that the set of vectors R with the dot product forms an inner product space. 10 Show that the set of polynomials P2(x)=ax+ bx +c forms an inner product space, where the inner product is defined as (P,Q) = P(x)Q(x)dx.