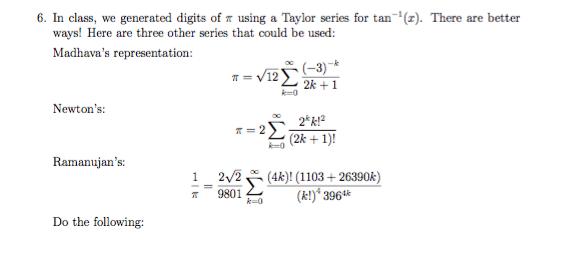
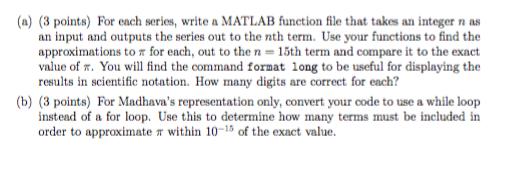
6. In class, we generated digits of using a Taylor series for tan (z). There are better ways! Here are three other series that could be used: Madhava's representation: Newton's: Ramanujan's: T 1 = 22 9801 Do the following: = 12 (3) 2k+1 =2 IM8 (2k + 1)! (4k)! (1103+26390k) (k!)*3964 (a) (3 points) For each series, write a MATLAB function file that takes an integer n as an input and outputs the series out to the nth term. Use your functions to find the approximations to for each, out to the n 15th term and compare it to the exact value of x. You will find the command format long to be useful for displaying the results in scientific notation. How many digits are correct for each? (b) (3 points) For Madhava's representation only, convert your code to use a while loop instead of a for loop. Use this to determine how many terms must be included in order to approximate r within 10-15 of the exact value.