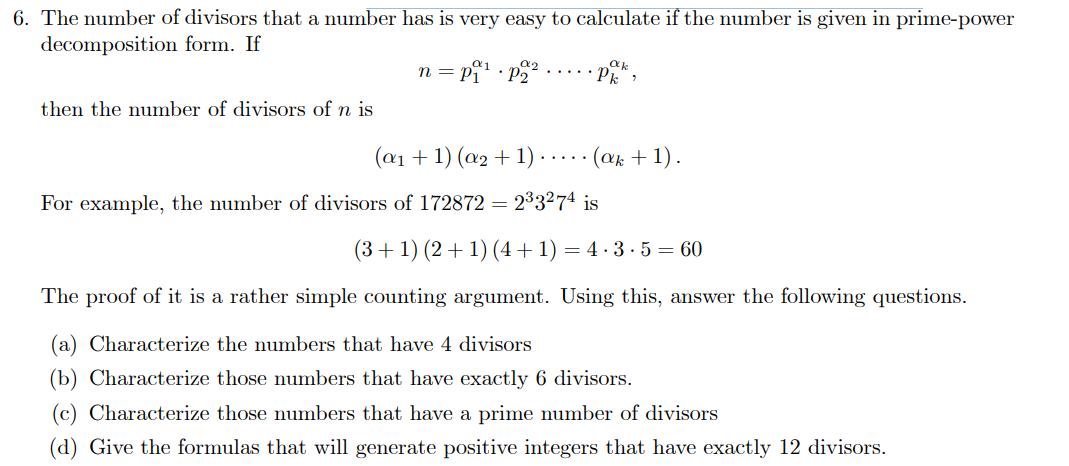
6. The number of divisors that a number has is very easy to calculate if the number is given in prime-power decomposition form. If n = p.p2 then the number of divisors of n is ak Pk 2 (a + 1) (a2 + 1).. (ak + 1). For example, the number of divisors of 172872 = 23274 is (3+1) (2+1) (4+1)=4.3.5 = 60 The proof of it is a rather simple counting argument. Using this, answer the following questions. (a) Characterize the numbers that have 4 divisors (b) Characterize those numbers that have exactly 6 divisors. (c) Characterize those numbers that have a prime number of divisors (d) Give the formulas that will generate positive integers that have exactly 12 divisors.