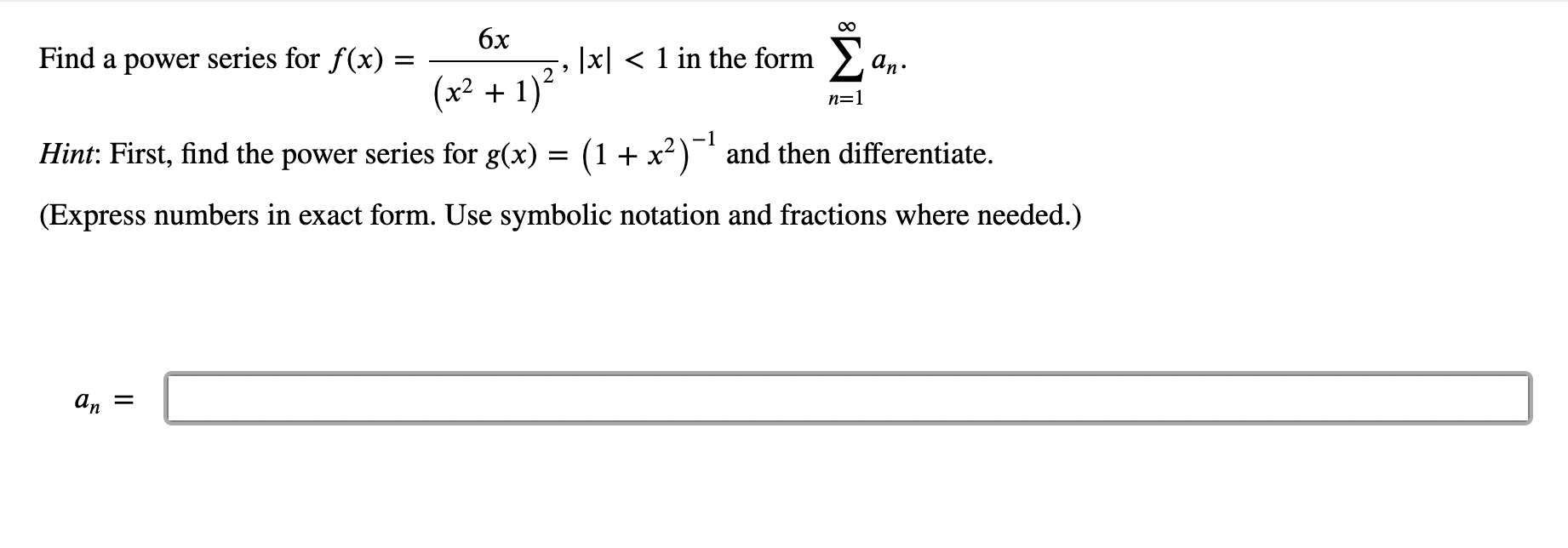
6x Find a power series for f(x): = |x| < 1 in the form an. (x + 1) n=1 -1 Hint: First, find the power series for g(x) = (1 + x) and then differentiate. (Express numbers in exact form. Use symbolic notation and fractions where needed.) an = 1 Expand the function in a power series anx" with center c = 0. Find anx". 5+4x n=0 (Express numbers in exact form. Use symbolic notation and fractions where needed. For alternating series, include a factor of the form (-1)" in your answer.) anxn = Determine the interval of convergence. (Give your answers as intervals in the form (*, *). Use symbol o for infinity, U for combining intervals, and appropriate type of parenthesis " (",") ", " [" or "] " depending on whether the interval is open or closed. Enter DNE if interval is empty. Express numbers in exact form. Use symbolic notation and fractions where needed.) xe Use the equation = || -x x" for |x| < 1 to expand the function 8 9-x in a power series with center c = = 0. n=0 (Use symbolic notation and fractions where needed.) 8 - x = n=0 I8 Determine the interval of convergence. (Use symbolic notation and fractions where needed. Give your answers as intervals in the form (*,*). Use the symbol for infinity, U for combining intervals, and an appropriate type of parenthesis "(", ")", "[" or "]" depending on whether the interval is open or closed.)