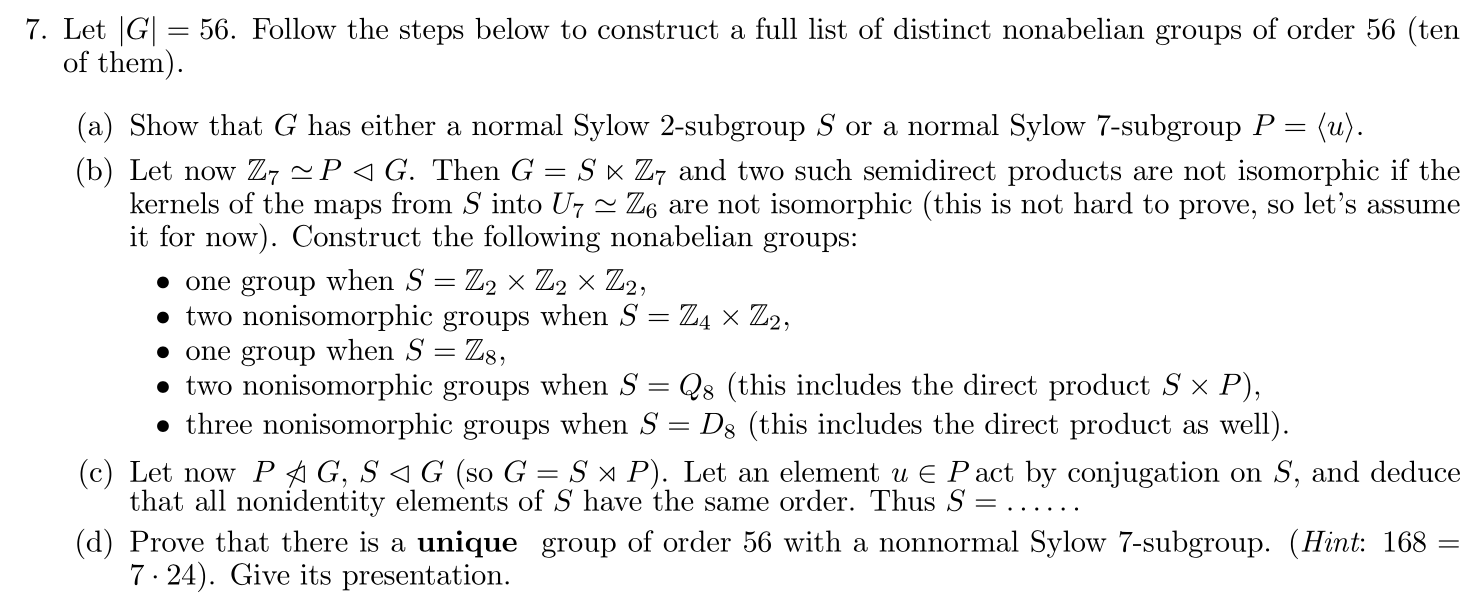
= 7. Let |G| = 56. Follow the steps below to construct a full list of distinct nonabelian groups of order 56 (ten of them). = (a) Show that G has either a normal Sylow 2-subgroup S or a normal Sylow 7-subgroup P = (u). (b) Let now Z7 - PAG. Then G S Z7 and two such semidirect products are not isomorphic if the kernels of the maps from S into U7 ~ Zo are not isomorphic (this is not hard to prove, so let's assume it for now). Construct the following nonabelian groups: one group when S = Z2 x Z2 x Z2, two nonisomorphic groups when S = Z4 x Z2, one group when S = Z8, two nonisomorphic groups when S = Q8 (this includes the direct product S x P), three nonisomorphic groups when S D8 (this includes the direct product as well). (c) Let now P&G, S - G (so G = S x P). Let an element u E Pact by conjugation on and deduce that all nonidentity elements of S have the same order. Thus S (d) Prove that there is a unique group of order 56 with a nonnormal Sylow 7-subgroup. (Hint: 168 7. 24). Give its presentation. = = = 7. Let |G| = 56. Follow the steps below to construct a full list of distinct nonabelian groups of order 56 (ten of them). = (a) Show that G has either a normal Sylow 2-subgroup S or a normal Sylow 7-subgroup P = (u). (b) Let now Z7 - PAG. Then G S Z7 and two such semidirect products are not isomorphic if the kernels of the maps from S into U7 ~ Zo are not isomorphic (this is not hard to prove, so let's assume it for now). Construct the following nonabelian groups: one group when S = Z2 x Z2 x Z2, two nonisomorphic groups when S = Z4 x Z2, one group when S = Z8, two nonisomorphic groups when S = Q8 (this includes the direct product S x P), three nonisomorphic groups when S D8 (this includes the direct product as well). (c) Let now P&G, S - G (so G = S x P). Let an element u E Pact by conjugation on and deduce that all nonidentity elements of S have the same order. Thus S (d) Prove that there is a unique group of order 56 with a nonnormal Sylow 7-subgroup. (Hint: 168 7. 24). Give its presentation. = =