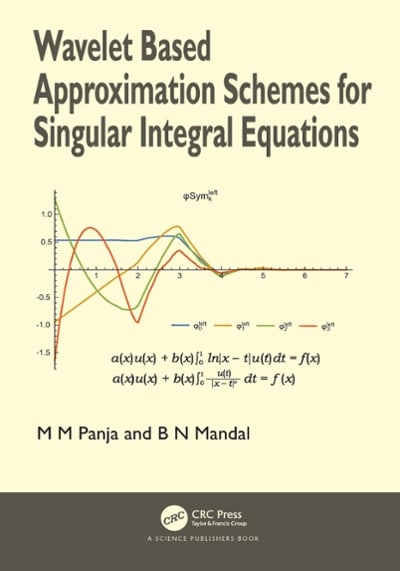
7 m terms . PLEASE help
1. (i) Define the terms "sample space", "event" and "random variable" and give an example of each. [10 points] (ii) What is an indicator random variable and what is the probability mass function of a discrete random variable ? [5 points] (ii) Define the conditional probability of an event and state Bayes Theorem. [5 points] (iv) Explain what is meant by "marginalization". [5 points] 2. Suppose we have two bags, labeled A and B. Bag A contains 3 white balls and 1 black ball, bag B contains 1 white ball and 3 black balls. We toss a fair coin and select bag A if it comes up heads and otherwise bag B. From the selected bag we now draw 5 balls, one after another, replacing each ball in the bag after it has been selected (the bag always contains 4 balls each time a ball is drawn). We observe 4 white balls and 1 black ball. What is the probability that we selected bag A ? Hint: use Bayes Rule. [20 points] 3. (i) Define the expected value of a random variable. Give a proof that the expected value is linear i.e. E[X+Y]=E[X]+E[Y] for random variables X and Y. [5 points] (ii) Define what it means for two random variables to be independent. Give a proof that when two random variables X and Y are independent then E[XY]=E[X]E[Y]. [5 points] (iii) Define the covariance and correlation of two random variables X and Y. [ 5 points] 4. (i) A bag contains 30 balls, of which 10 are red and the other 20 blue. Suppose you take out 8 balls from this bag, with replacement. What is the probability that among the 8 balls in this sample exactly 3 are red and 5 are blue? [5 points] (ii) Now suppose that the balls are taken out of the bag without replacement. What is the probability that out of 8 balls exactly 3 are red and 5 are blue? [10 points]1. (1) Define the terms "sample space", "event" and "random variable" and give an example of each. [10 points] (ii) What is an indicator random variable and what is the probability mass function of a discrete random variable ? [5 points] (iii) Define the conditional probability of an event and state Bayes Theorem. [5 points] (iv) Explain what is meant by "marginalization". [5 points] 2. Suppose we have two bags, labeled A and B. Bag A contains 3 white balls and 1 black ball, bag B contains 1 white ball and 3 black balls. We toss a fair coin and select bag A if it comes up heads and otherwise bag B. From the selected bag we now draw 5 balls, one after another, replacing each ball in the bag after it has been selected (the bag always contains 4 balls each time a ball is drawn). We observe 4 white balls and 1 black ball. What is the probability that we selected bag A 7 Hint: use Bayes Rule. [20 points] 3. (1) Define the expected value of a random variable. Give a proof that the expected value is linear i.e. E[X+Y]=E[X]+E[Y] for random variables X and Y. [5 points] (ii) Define what it means for two random variables to be independent. Give a proof that when two random variables X and Y are independent then E[XY]=E[XJE[Y]. [5 points] (ii) Define the covariance and correlation of two random variables X and Y. [ 5 points] 4. (1) A bag contains 30 balls, of which 10 are red and the other 20 blue. Suppose you take out 8 balls from this bag, with replacement. What is the probability that among the 8 balls in this sample exactly 3 are red and 5 are blue? [5 points] (ii) Now suppose that the balls are taken out of the bag without replacement. What is the probability that out of 8 balls exactly 3 are red and 5 are blue? [10 points]