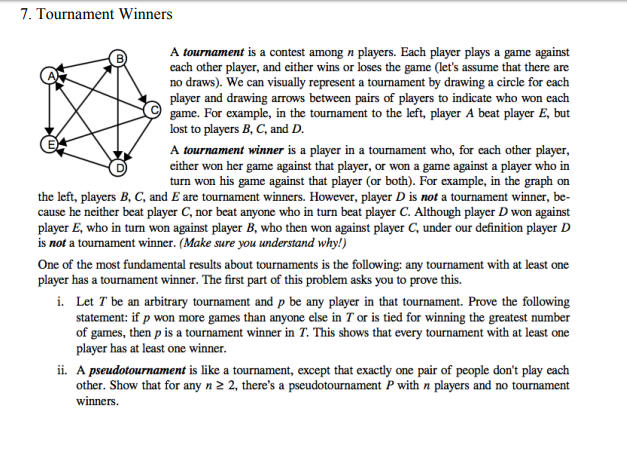
7. Tournament Winners A tournament is a contest among n players. Each player plays a game against each other player, and either wins or loses the game (let's assume that there are no draws). We can visually represent a tournament by drawing a circle for each player and drawing arrows between pairs of players to indicate who won each game. For example, in the tournament to the left, player A beat player E, but lost to players B, C, and D. A tournament winner is a player in a tournament who, for each other player, either won her game against that player, or won a game against a player who in turn won his game against that player (or both). For example, in the graph on the left, players B, C, and E are tournament winners. However, player D is not a tournament winner, be- cause he neither beat player C, nor beat anyone who in turn beat player C. Although player D won against player E, who in turn won against player B, who then won against player C, under our definition player D is not a tournament winner. (Make sure you understand why!) One of the most fundamental results about tournaments is the followin player has a tournament winner. The first part of this problem asks you to prove this. any tournament with at least one i. Let T be an arbitrary tournament and p be any player in that tournament. Prove the following statement: if p won more games than anyone else in T or is tied for winning the greatest number t with at least one in T. This shows that every tournamen of games, then p is a tournament winner player has at least one winner ii. A pseudotournament is like a tournament, except that exactly one pair of people don't play each other. Show that for any n 2 2, there's a pseudotournament P with n players and no tournament winners. 7. Tournament Winners A tournament is a contest among n players. Each player plays a game against each other player, and either wins or loses the game (let's assume that there are no draws). We can visually represent a tournament by drawing a circle for each player and drawing arrows between pairs of players to indicate who won each game. For example, in the tournament to the left, player A beat player E, but lost to players B, C, and D. A tournament winner is a player in a tournament who, for each other player, either won her game against that player, or won a game against a player who in turn won his game against that player (or both). For example, in the graph on the left, players B, C, and E are tournament winners. However, player D is not a tournament winner, be- cause he neither beat player C, nor beat anyone who in turn beat player C. Although player D won against player E, who in turn won against player B, who then won against player C, under our definition player D is not a tournament winner. (Make sure you understand why!) One of the most fundamental results about tournaments is the followin player has a tournament winner. The first part of this problem asks you to prove this. any tournament with at least one i. Let T be an arbitrary tournament and p be any player in that tournament. Prove the following statement: if p won more games than anyone else in T or is tied for winning the greatest number t with at least one in T. This shows that every tournamen of games, then p is a tournament winner player has at least one winner ii. A pseudotournament is like a tournament, except that exactly one pair of people don't play each other. Show that for any n 2 2, there's a pseudotournament P with n players and no tournament winners