7.22. The one-year LIBOR rates is 3%, and the LIBOR forward rate for the 1- to 2-year period is 3.2%, respectively. The three-year swap rate for a swap with annual payments is 3.2%. What is the LIBOR forward rate for the 2- to 3-year period if OIS zero rates for maturities of one, two, and three years are 2.5%, 2.7%, and 2.9%, respectively. What is the value of a three-year swap where 4% is received and LIBOR is paid on a principal of $100 million. All rates are annually compounded 7.23. In an interest rate swap, a financial institution has agreed to pay 3.6% per annum and to receive three-month LIBOR in return on a notional principal of $100 million with payments being exchanged every three months. The swap has a remaining life of 14 months. Three-month forward LIBOR for all maturities is currently 4% per annum. The three-month LIBOR rate one month ago was 3.2% per annum. OIS rates for all maturities are currently 3.8% with continuous compounding. All other rates are com- pounded quarterly. What is the value of the swap? 7.24. Company A, a British manufacturer, wishes to borrow U.S. dollars at a fixed rate of interest. Company B, a U.S. multinational, wishes to borrow sterling at a fixed rate of interest. They have been quoted the following rates per annum: Sterling U.S. Dollars Company A 11.0% 7.0% Company B 10.6% 6.2%

Rates have been adjusted for differential tax effects.) Design a swap that will net a bank, acting as intermediary, 10 basis points per annum and that will produce a gain of 15 basis points per annum for each of the two companies. 7.25. Suppose that the term structure of risk-free interest rates is flat in the United States and Australia. The USD interest rate is 7% per annum and the AUD rate is 9% per annum. The current value of the AUD is 0.62 USD. Under the terms of a swap agreement, a financial institution pays 8% per annum in AUD and receives 4% per annum in USD. The principals in the two currencies are $12 million USD and 20 million AUD. Payments are exchanged every year, with one exchange having just taken place. The swap will last two more years. What is the value of the swap to the financial institution? Assume all interest rates are continuously compounded.

7.26. The five-year swap rate when cash flows are exchanged semiannually is 4%. A company wants a swap where it receives payments at 4.2% per annum on a principal of $10 million. The OIS zero curve is flat at 3.6%. How much should a derivatives dealer charge the company. All rates are expressed with semiannual compounding. (Ignore bid-offer spreads.) Suppose that the principal assigned to the senior, mezzanine, and equity tranches is 70%, 20%, and 10% for both the ABS and the ABS CDO in Figure 8.3. What difference does this make to Table 8.1? 8.17. ''Resecuritization was a badly flawed idea. AAA tranches created from the mezzanine tranches of ABSs are bound to have a higher probability of default than the AAA-rated tranches of ABSs.'' Discuss this point of view. 8.18. Suppose that mezzanine tranches of the ABS CDOs, similar to those in Figure 8.3, are resecuritized to form what is referred to as a ''CDO squared.'' As in the case of tranches created from ABSs in Figure 8.3, 65% of the principal is allocated to a AAA tranche, 25% to a BBB tranche, and 10% to the equity tranche. How high does the loss percentage

question 1

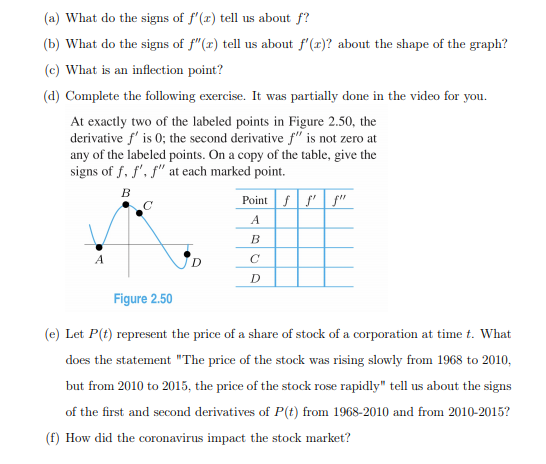
(a) What do the signs of f'(x) tell us about f? (b) What do the signs of f"(x) tell us about f'(x)? about the shape of the graph? (c) What is an inflection point? (d) Complete the following exercise. It was partially done in the video for you. At exactly two of the labeled points in Figure 2.50, the derivative f' is 0; the second derivative f" is not zero at any of the labeled points. On a copy of the table, give the signs of f, f', f" at each marked point. B C Point " A B D C D Figure 2.50 (e) Let P(t) represent the price of a share of stock of a corporation at time t. What does the statement "The price of the stock was rising slowly from 1968 to 2010, but from 2010 to 2015, the price of the stock rose rapidly" tell us about the signs of the first and second derivatives of P(t) from 1968-2010 and from 2010-2015? (f) How did the coronavirus impact the stock market?