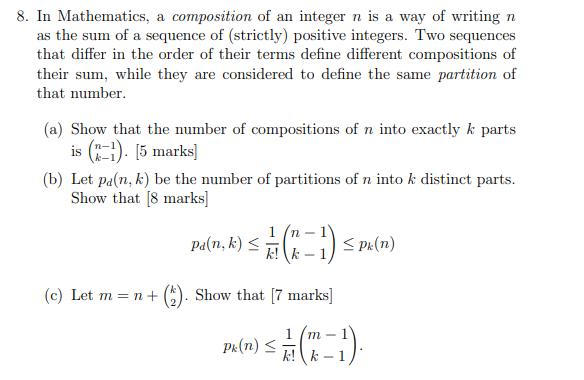
8. In Mathematics, a composition of an integer n is a way of writing n as the sum of a sequence of (strictly) positive integers. Two sequences that differ in the order of their terms define different compositions of their sum, while they are considered to define the same partition of that number. (a) Show that the number of compositions of n into exactly k parts is (-1). [5 marks] (b) Let pa(n, k) be the number of partitions of n into k distinct parts. Show that [8 marks] 1 n- pa(n, k) < (^= 1) = Pk (n) k! k- (c) Let m = n + (). Show that [7 marks] Pu(n) / 1 (n=1). k! k -1