Question
9 Consider a continuous-time Markov chain {X(t)} whose jump chain is the success run chain from Problem 16. Give a condition on the holding time
9 Consider a continuous-time Markov chain {X(t)} whose jump chain is the success run
chain from Problem 16. Give a condition on the holding time parameters ?(k), k =
0, 1, ... guaranteeing that {X(t)} has a stationary distribution (remember the previous
problem).
40 Consider a linear birth-death process where the individual birth rate is ? = 1, the
individual death rate is = 3 and there is constant immigration into the population
according to a Poisson process with rate ?. (a) State the rate diagram and the generator.
(b) Suppose that there are currently 10 individuals in the population. What is the
probability that the population size increases to 11 before it decreases to 9? (c) Suppose
that ? = 1 and that the population just became extinct. What is the expected time until
it becomes extinct again?
41 In the previous problem suppose that an immigrating individual joins the population only
if it is extinct, and otherwise leaves. Find the rate diagram, generator, and stationary
distribution.
42 Consider state 0 in a birth-death process with stationary distribution ?. Under stationary
conditions we ought to have the balance equation ?0?0 = ?11 ("rate in equals rate
out"), which is also precisely the first equation of ? G = 0. (a) Suggest how to formulate
balance equations for any three states k ?1, k, and k + 1, and show that these equations
are the same as ?G = 0. (b) Describe how the equation?G = 0 has an interpretation as
balance equations for any continuous-time Markov chain, not just birth-death processes.
43 Consider an M/M/1/r queue in equilibrium where r = 5 and the service rate equals
the arrival rate. (a) What is the proportion of lost customers? (b) How does this change
if the service rate is doubled?
44 Consider a queueing system where there is one server and no room to wait in line (i.e.,
an M/M/1/1 queue). Further suppose that the arrival rate ? and the service rate are
equal. Under stationary conditions, find the proportion of customers that are lost (a) in
this system, (b) if the service rate is doubled, (c) if one customer can wait in line, (d) if
a second server is added
Consider a continuous-time Markov chain where state i is absorbing. How should the
ith row of the generator G be defined?
34 Consider the ON/OFF system in Example 8.4.1. State the backward and forward equations and solve the forward equations. (Why are these easier to solve than the backward
equations?) What happens as t ? ??
35 Birds arrive at four bird feeders according to a Poisson process with rate one bird per
minute. If all feeders are occupied, an arriving bird leaves, but otherwise it occupies a
feeder and eats for a time that has an exponential distribution with mean one minute.
Consider this as a Markov chain where a "state" is the number of occupied feeders. The
rate diagram is given below. (a) Explain the rates in the diagram. (b) Find the generator
G. (c) If three feeders are occupied, what is the expected time until this changes? (d)
If all feeders are occupied, what is the probability that a bird arrives before a feeder
becomes free?
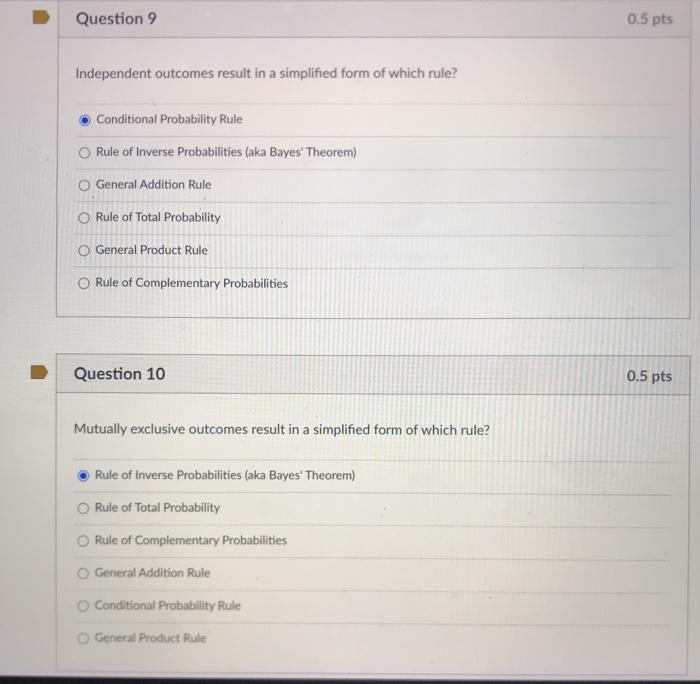


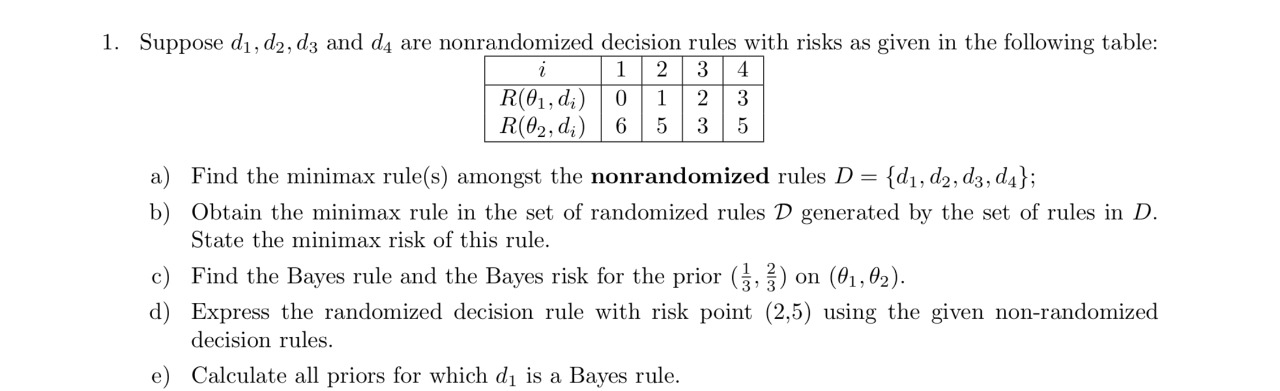
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


