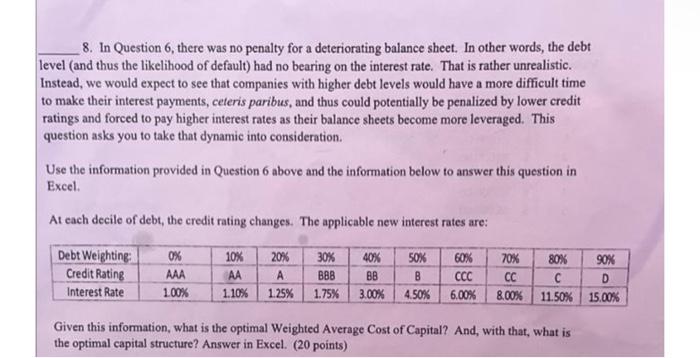
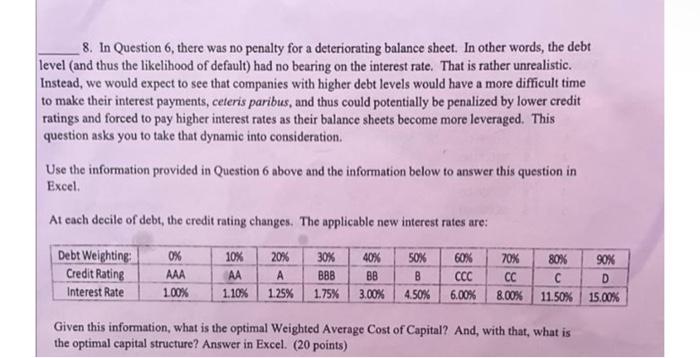
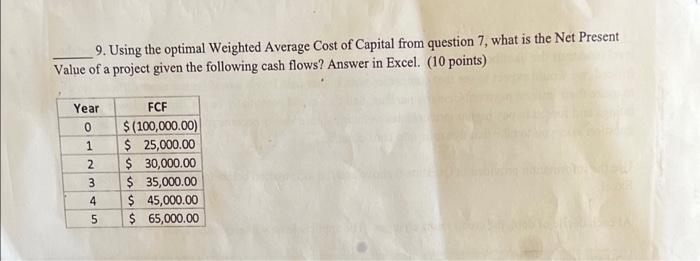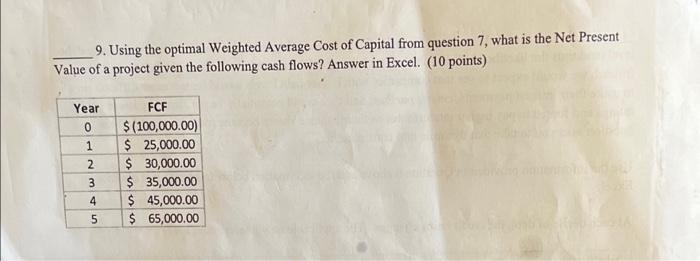9. Using the optimal Weighted Average Cost of Capital from question 7, what is the Net Present Value of a project given the following cash flows? Answer in Excel. (10 points) Year 0 1 2 3 Nm FCF $(100,000.00) $ 25,000.00 $ 30,000.00 $ 35,000.00 $ 45,000.00 $ 65,000.00 4 5 8. In Question 6, there was no penalty for a deteriorating balance sheet. In other words, the debt level (and thus the likelihood of default) had no bearing on the interest rate. That is rather unrealistic. Instead, we would expect to see that companies with higher debt levels would have a more difficult time to make their interest payments, ceteris paribus, and thus could potentially be penalized by lower credit ratings and forced to pay higher interest rates as their balance sheets become more leveraged. This question asks you to take that dynamic into consideration. Use the information provided in Question 6 above and the information below to answer this question in Excel At cach decile of debt, the credit rating changes. The applicable new interest rates are: Debt Weighting Credit Rating Interest Rate 0% AAA 1.00% 10% AA 1.10% 20% A 1.25% 30% BBB 1.75% 40% BB 3.00% 50% B 4.50% 60% CCC 70% 80% 90% CC D 8.00% 11.50% 15.00% 6.00% Given this information, what is the optimal Weighted Average Cost of Capital? And, with that, what is the optimal capital structure? Answer in Excel. (20 points) 9. Using the optimal Weighted Average Cost of Capital from question 7, what is the Net Present Value of a project given the following cash flows? Answer in Excel. (10 points) Year 0 1 N 2 FCF $(100,000.00) $ 25,000.00 $ 30,000.00 $ 35,000.00 $ 45,000.00 $ 65,000.00 3 4 5 8. In Question 6, there was no penalty for a deteriorating balance sheet. In other words, the debt level (and thus the likelihood of default) had no bearing on the interest rate. That is rather unrealistic. Instead, we would expect to see that companies with higher debt levels would have a more difficult time to make their interest payments, ceteris paribus, and thus could potentially be penalized by lower credit ratings and forced to pay higher interest rates as their balance sheets become more leveraged. This question asks you to take that dynamic into consideration. Use the information provided in Question 6 above and the information below to answer this question in Excel At cach decile of debt, the credit rating changes. The applicable new interest rates are: 60% Debt Weighting Credit Rating Interest Rate 0% AAA 1.00% 10% AA 1.10% 20% A 1.25% 30% BBB 1.75% 40% BB 3.00% 50% B 4.50% CCC 6.00% 70% 80% 90% CC D 8.00% 11.50% 15.00% Given this information, what is the optimal Weighted Average Cost of Capital? And, with that, what is the optimal capital structure? Answer in Excel. (20 points) 9. Using the optimal Weighted Average Cost of Capital from question 7, what is the Net Present Value of a project given the following cash flows? Answer in Excel. (10 points) Year 0 1 2 3 Nm FCF $(100,000.00) $ 25,000.00 $ 30,000.00 $ 35,000.00 $ 45,000.00 $ 65,000.00 4 5 8. In Question 6, there was no penalty for a deteriorating balance sheet. In other words, the debt level (and thus the likelihood of default) had no bearing on the interest rate. That is rather unrealistic. Instead, we would expect to see that companies with higher debt levels would have a more difficult time to make their interest payments, ceteris paribus, and thus could potentially be penalized by lower credit ratings and forced to pay higher interest rates as their balance sheets become more leveraged. This question asks you to take that dynamic into consideration. Use the information provided in Question 6 above and the information below to answer this question in Excel At cach decile of debt, the credit rating changes. The applicable new interest rates are: Debt Weighting Credit Rating Interest Rate 0% AAA 1.00% 10% AA 1.10% 20% A 1.25% 30% BBB 1.75% 40% BB 3.00% 50% B 4.50% 60% CCC 70% 80% 90% CC D 8.00% 11.50% 15.00% 6.00% Given this information, what is the optimal Weighted Average Cost of Capital? And, with that, what is the optimal capital structure? Answer in Excel. (20 points) 9. Using the optimal Weighted Average Cost of Capital from question 7, what is the Net Present Value of a project given the following cash flows? Answer in Excel. (10 points) Year 0 1 N 2 FCF $(100,000.00) $ 25,000.00 $ 30,000.00 $ 35,000.00 $ 45,000.00 $ 65,000.00 3 4 5 8. In Question 6, there was no penalty for a deteriorating balance sheet. In other words, the debt level (and thus the likelihood of default) had no bearing on the interest rate. That is rather unrealistic. Instead, we would expect to see that companies with higher debt levels would have a more difficult time to make their interest payments, ceteris paribus, and thus could potentially be penalized by lower credit ratings and forced to pay higher interest rates as their balance sheets become more leveraged. This question asks you to take that dynamic into consideration. Use the information provided in Question 6 above and the information below to answer this question in Excel At cach decile of debt, the credit rating changes. The applicable new interest rates are: 60% Debt Weighting Credit Rating Interest Rate 0% AAA 1.00% 10% AA 1.10% 20% A 1.25% 30% BBB 1.75% 40% BB 3.00% 50% B 4.50% CCC 6.00% 70% 80% 90% CC D 8.00% 11.50% 15.00% Given this information, what is the optimal Weighted Average Cost of Capital? And, with that, what is the optimal capital structure? Answer in Excel. (20 points)