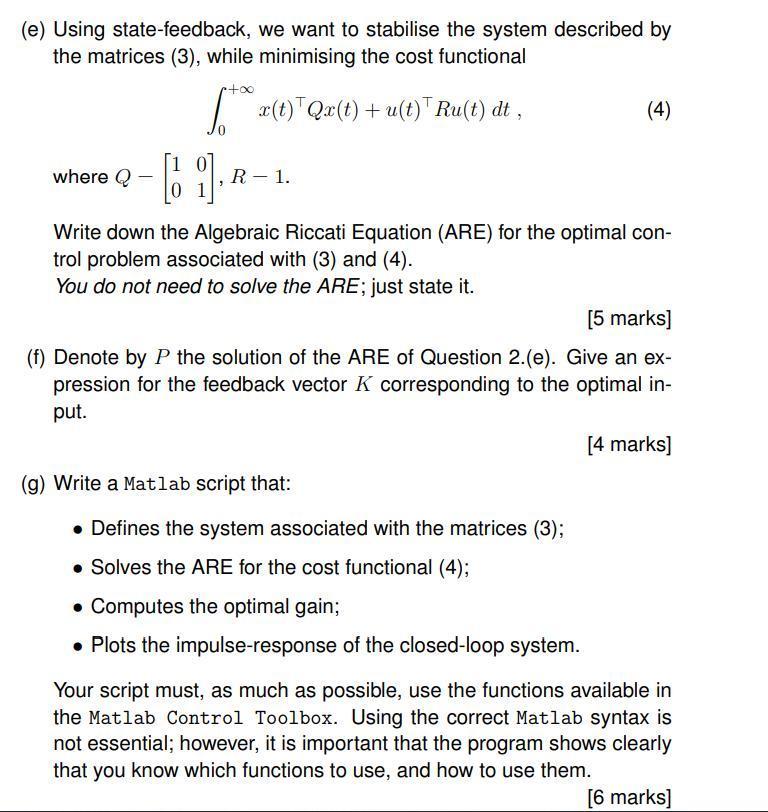
(e) Using state-feedback, we want to stabilise the system described by the matrices (3), while minimising the cost functional r+ x(t) Qx(t) + u(t) Ru(t) dt, where Q -61 Write down the Algebraic Riccati Equation (ARE) for the optimal con- trol problem associated with (3) and (4). You do not need to solve the ARE; just state it. R 1. (4) [5 marks] (f) Denote by P the solution of the ARE of Question 2.(e). Give an ex- pression for the feedback vector K corresponding to the optimal in- put. (g) Write a Matlab script that: Defines the system associated with the matrices (3); Solves the ARE for the cost functional (4); Computes the optimal gain; Plots the impulse-response of the closed-loop system. [4 marks] Your script must, as much as possible, use the functions available in the Matlab Control Toolbox. Using the correct Matlab syntax is not essential; however, it is important that the program shows clearly that you know which functions to use, and how to use them. [6 marks]