$A=\left[\begin{array}{ccccc}1& ?& ?& ?& ?\\ \frac{1}{h^2}& -\frac{2}{h^2}& \frac{1}{h^2}& ?& ?\\ ?& \frac{1}{h^2}& -\frac{2}{h^2}& \frac{1}{h^2}& ?\\ ?& \text{d}\text{d}\text{o}\text{t}\text{s}& \text{d}\text{d}\text{o}\text{t}\text{s}& \text{d}\text{d}\text{o}\text{t}\text{s}& ?\\ ?& \frac{1}{h^2}& -\frac{2}{h^2}& \frac{1}{h^2}& ?\\ ?& 1& ?& ?& ?\end{array}\right]$

a$)$ Code a function for A for a given number of steps $N.$ The easiest way to do this is to define three

column vectors containing the entries of the main diagonal $(N+1$ entries$),$ subdiagonal $(N$ entries$)$ and

superdiagonal $(N$ entries$).$ The np$.$ones command is useful here:

These vectors can be combined into a tridiagonal matrix using the np$.$diag command: $\frac{?}{?}$# superdiagonal $(N$ entries$)$

sup$=...$

These vectors can be combined into a tridiagonal matrix using the $np.$ diag command:

$\frac{?}{?}$# create tridiagonal matrix

$A=np.$diag$(main)+np.$diag$(sup,1)+np.$diag$(sub,-1)$

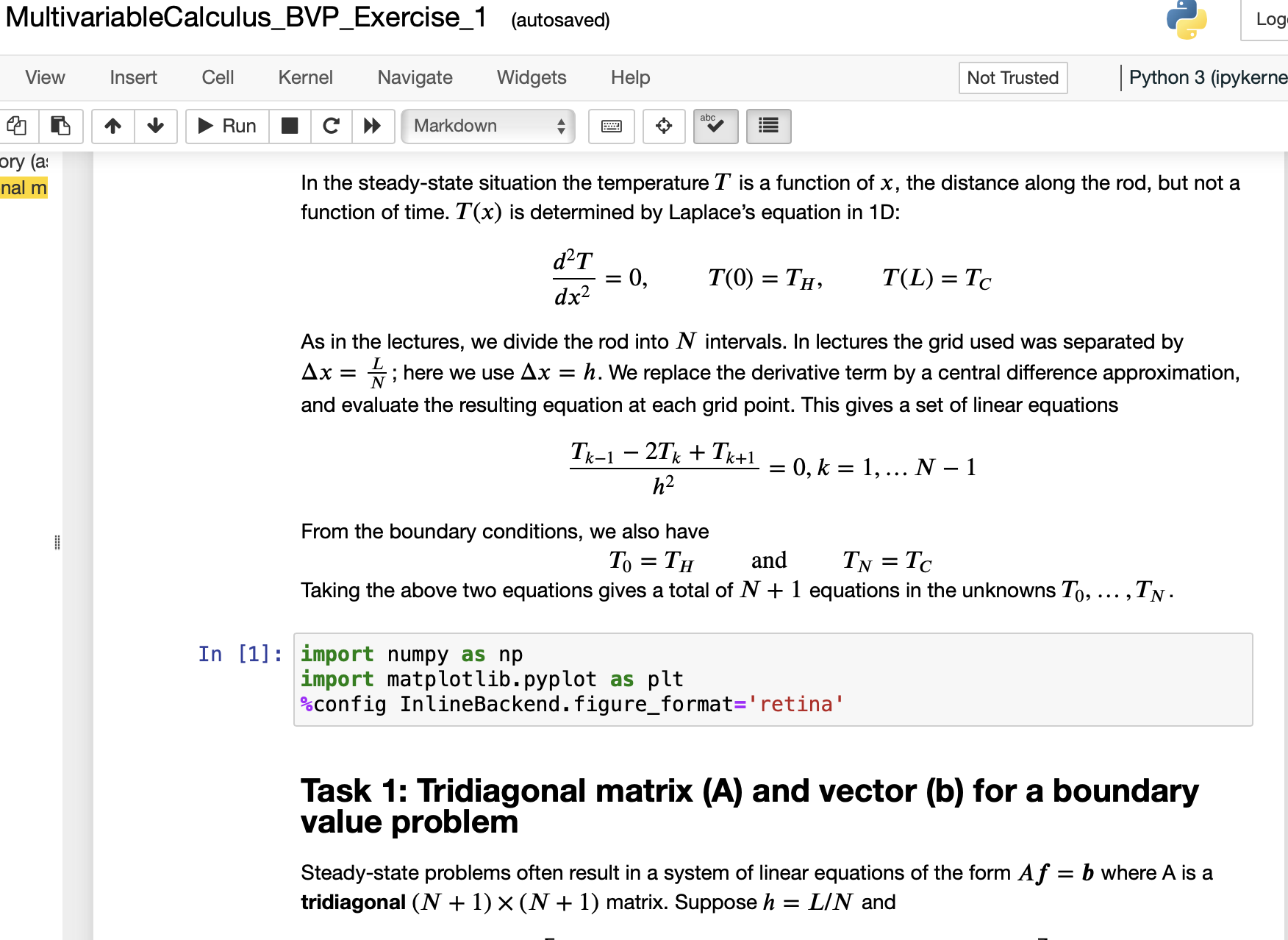
Your function should take arguments $N,L$ and $h,$ and return the matrix $A.$In the steady$-$state situation the temperature $T$ is a function of $x,$ the distance along the rod, but not a

function of time. $T(x)$ is determined by Laplace's equation in $1$D:

$\frac{d^{2}T}{d{x}^2}=0,T(0)=T_H,T(L)=T_C$

As in the lectures, we divide the rod into $N$ intervals. In lectures the grid used was separated by

$x=\frac{L}{N}$; here we use $x=h.$ We replace the derivative term by a central difference approximation,

and evaluate the resulting equation at each grid point. This gives a set of linear equations

$\frac{T_{k-1}-2T_k+T_{k+1}}{h^2}=0,k=1,$dotsN$-1$

From the boundary conditions, we also have

$T_0=T_H,$ and $,T_N=T_C$

Taking the above two equations gives a total of $N+1$ equations in the unknowns $T_0,$dots,$T_N.$

In $[1]$: import numpy as np

import matplotlib.pyplot as plt

$\%$config InlineBackend.figure$\_$format$=$'retina'

Task $1$: Tridiagonal matrix $($A$)$ and vector $($b$)$ for a boundary

value problem

Steady$-$state problems often result in a system of linear equations of the form $Af=b$ where $A$ is a

tridiagonal $(N+1)(N+1)$ matrix. Suppose $h=\frac{L}{N}$ and