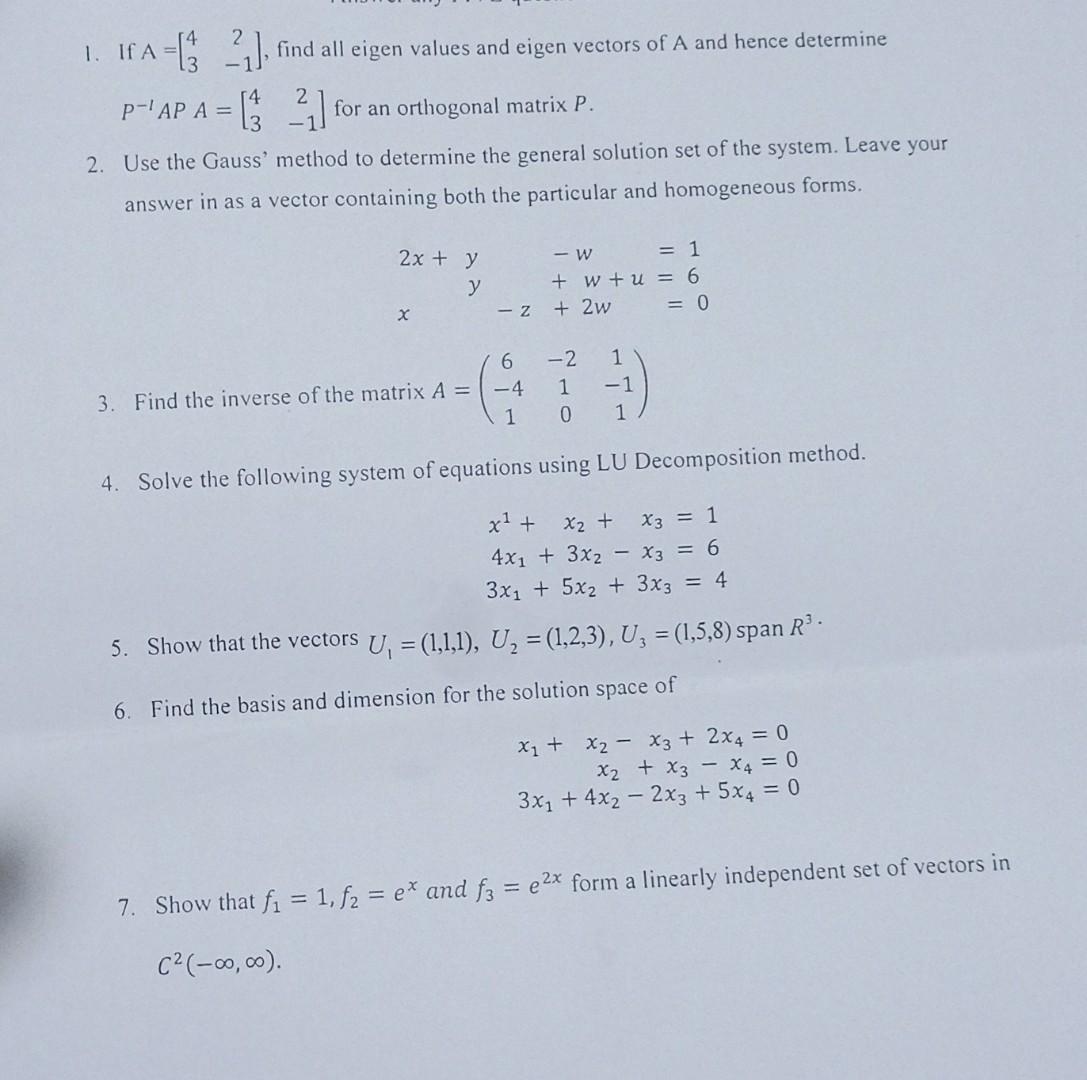
A = [3 2], find all eigen values and eigen vectors of A and hence determine =[] for an orthogonal matrix P. 2. Use the Gauss' method to determine the general solution set of the system. Leave your answer in as a vector containing both the particular and homogeneous forms. 1. If A P-AP A = 2x + y y x -z 6 3. Find the inverse of the matrix A = -4 - W = 1 +w+u = 6 + 2w = 0 1 1 -1 -2 1 0 1 4. Solve the following system of equations using LU Decomposition method. x + X + X3 = 1 4x + 3x2 - X3 = 6 3x + 5x2 + 3x3 = 4 5. Show that the vectors U = (1,1,1), U = (1,2,3), U = (1,5,8) span R - 6. Find the basis and dimension for the solution space of X + X - x3 + 2x4 = 0 X2 X3 X4 = 0 3x + 4x2 - 2x3 + 5x4 = 0 7. Show that f = 1, f = ex and f3 = e2x form a linearly independent set of vectors in C (-0,00).