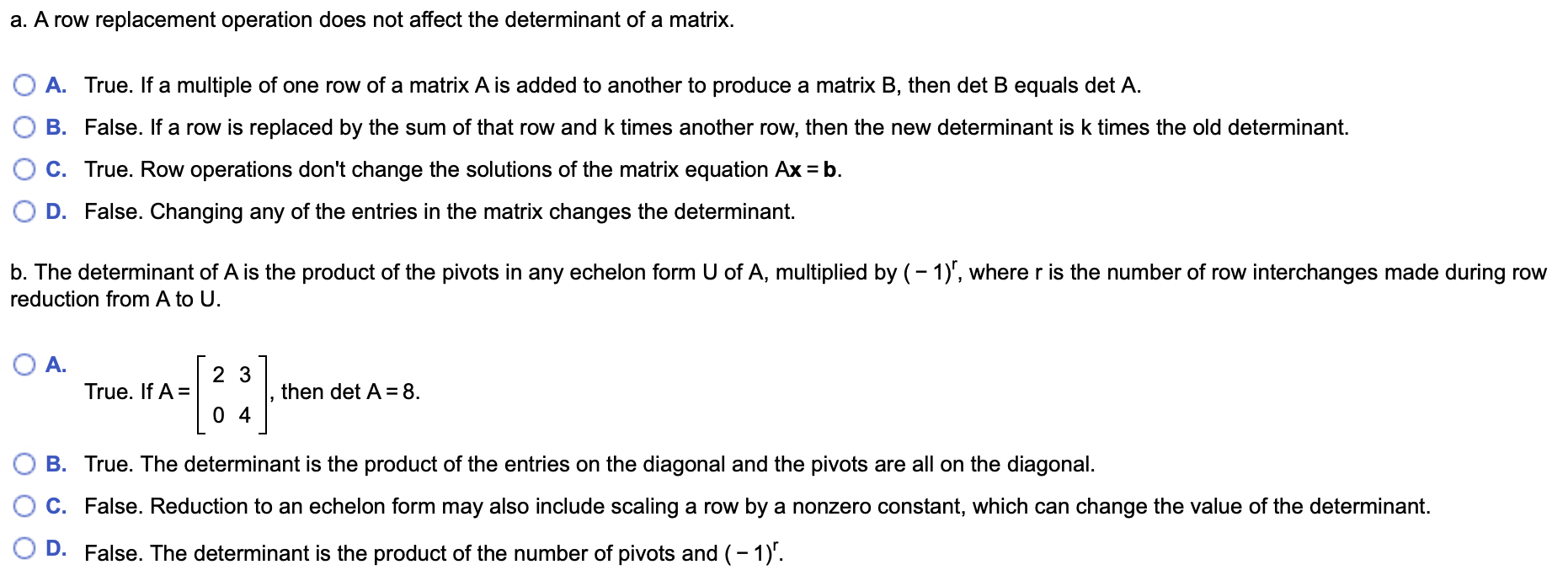
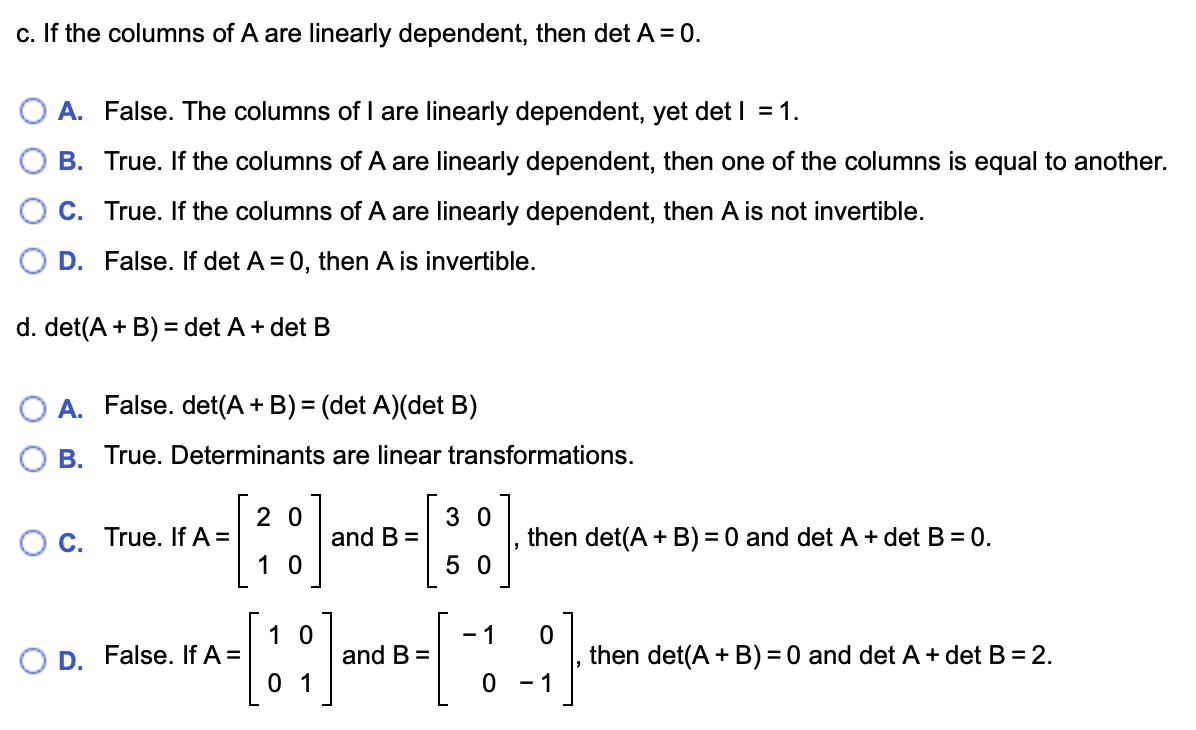
a. A row replacement operation does not affect the determinant of a matrix. O A. True. If a multiple of one row of a matrix A is added to another to produce a matrix B, then det B equals det A. B. False. If a row is replaced by the sum of that row and k times another row, then the new determinant is k times the old determinant. C. True. Row operations don't change the solutions of the matrix equation Ax = b. D. False. Changing any of the entries in the matrix changes the determinant. b. The determinant of A is the product of the pivots in any echelon form U of A, multiplied by (-1)", where r is the number of row interchanges made during row reduction from A to U. A. True. If A = 23 04 then det A = 8. B. True. The determinant is the product of the entries on the diagonal and the pivots are all on the diagonal. C. False. Reduction to an echelon form may also include scaling a row by a nonzero constant, which can change the value of the determinant. D. False. The determinant is the product of the number of pivots and (-1)". c. If the columns of A are linearly dependent, then det A = 0. A. False. The columns of I are linearly dependent, yet det I = 1. B. True. If the columns of A are linearly dependent, then one of the columns is equal to another. C. True. If the columns of A are linearly dependent, then A is not invertible. D. False. If det A = 0, then A is invertible. d. det(A + B) = det A + det B A. False. det(A + B) = (det A)(det B) B. True. Determinants are linear transformations. O c. True. If A = D. False. If A = 20 10 10 0 1 and B = and B = 30 50 - 1 then det(A + B) = 0 and det A + det B = 0. 0 0 - 1 then det(A + B) = 0 and det A + det B = 2.