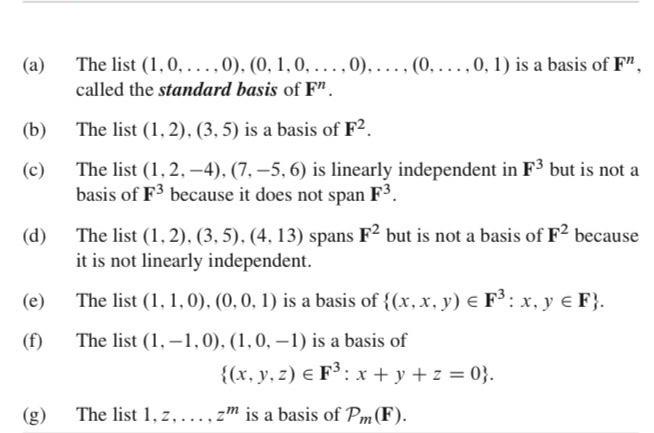
(a) (b) (c) The list (1,0,..., 0), (0, 1, 0, . . . , 0), . . . , (0, . . . , 0, 1) is a basis of F", called the standard basis of Fn. The list (1, 2), (3, 5) is a basis of F2. The list (1,2,-4), (7,-5, 6) is linearly independent in F but is not a basis of F3 because it does not span F. (d) The list (1,2). (3.5). (4, 13) spans F2 but is not a basis of F because it is not linearly independent. The list (1, 1,0), (0, 0, 1) is a basis of {(x, x, y) F : x, y F}. The list (1,-1,0), (1, 0, -1) is a basis of (e) (f) {(x, y, z) F: x + y + z = 0}. The list 1, 2,..., zm is a basis of Pm (F).