Answered step by step
Verified Expert Solution
Question
1 Approved Answer
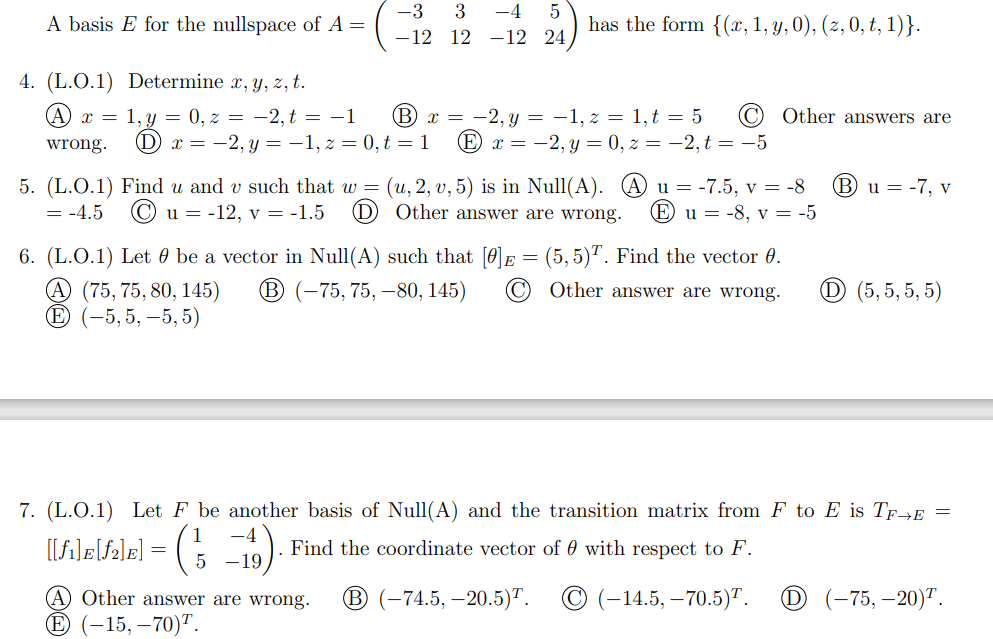
A basis E for the nullspace of A=([-3,3,-4,5],[-12,12,-12,24]) has the form {(x,1,y,0),(z,0,t,1)} . (L.O.1) Determine x,y,z,t . (A) x=1,y=0,z=-2,t=-1 (B) x=-2,y=-1,z=1,t=5 (C) Other
A basis
Efor the nullspace of
A=([-3,3,-4,5],[-12,12,-12,24])has the form
{(x,1,y,0),(z,0,t,1)}.\ (L.O.1) Determine
x,y,z,t.\ (A)
x=1,y=0,z=-2,t=-1\ (B)
x=-2,y=-1,z=1,t=5\ (C) Other answers are\ wrong.\ (D)
x=-2,y=-1,z=0,t=1\ (E)
x=-2,y=0,z=-2,t=-5\ (L.O.1) Find
uand
vsuch that
w=(u,2,v,5)is in
\\\\Nu ll(A). (A)
u=-7.5,v=-8\ (B)
u=-7,v\
=-4.5\ (C)
u=-12,v=-1.5\ (D) Other answer are wrong.\ (E)
u=-8,v=-5\ (L.O.1) Let
\\\\theta be a vector in
\\\\Nu ll(A)such that
[\\\\theta ]_(E)=(5,5)^(T). Find the vector
\\\\theta .\ (A)
(75,75,80,145)\ (B)
(-75,75,-80,145)\ (C) Other answer are wrong.\ (D)
(5,5,5,5)\ (E)
(-5,5,-5,5)\ (L.O.1) Let
Fbe another basis of
\\\\Nu ll(A)and the transition matrix from
Fto
Eis
T_(F->E)=\
[[f_(1)]_(E)[f_(2)]_(E)]=([1,-4],[5,-19]). Find the coordinate vector of
\\\\theta with respect to
F.\ (A) Other answer are wrong.\ (B)
(-74.5,-20.5)^(T).\ (C)
(-14.5,-70.5)^(T).\ (D)
(-75,-20)^(T).\ (E)
(-15,-70)^(T).
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


