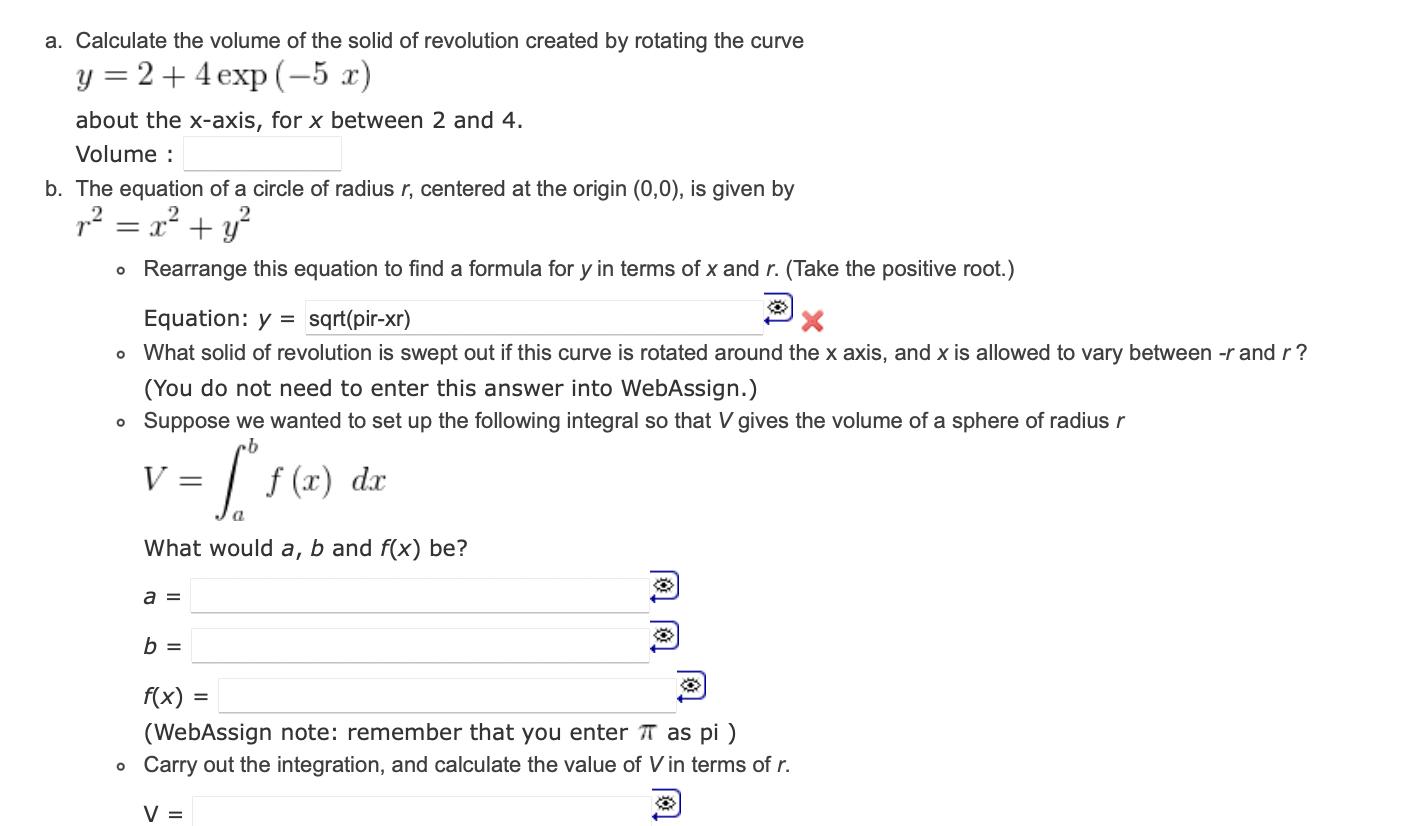
a. Calculate the volume of the solid of revolution created by rotating the curve y=2+4 exp(-5 x) about the x-axis, for x between 2 and 4. Volume: b. The equation of a circle of radius r, centered at the origin (0,0), is given by 2 2 r = x + y Rearrange this equation to find a formula for y in terms of x and r. (Take the positive root.) Equation: y = sqrt(pir-xr) What solid of revolution is swept out if this curve is rotated around the x axis, and x is allowed to vary between -r and r? (You do not need to enter this answer into WebAssign.) Suppose we wanted to set up the following integral so that V gives the volume of a sphere of radius r V = 1(x) f(x) dx What would a, b and f(x) be? a = b = f(x) = (WebAssign note: remember that you enter as pi ) Carry out the integration, and calculate the value of Vin terms of r. V =