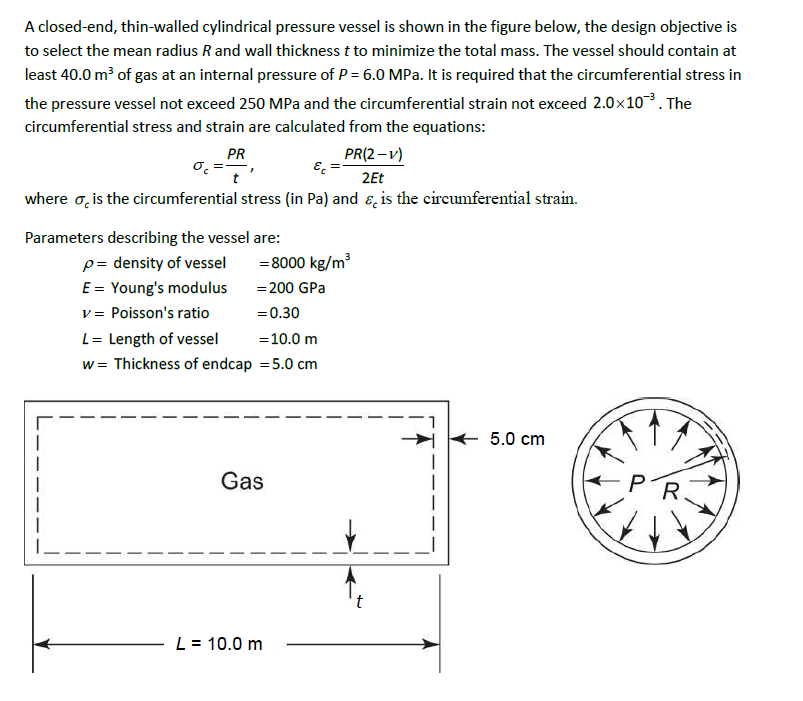
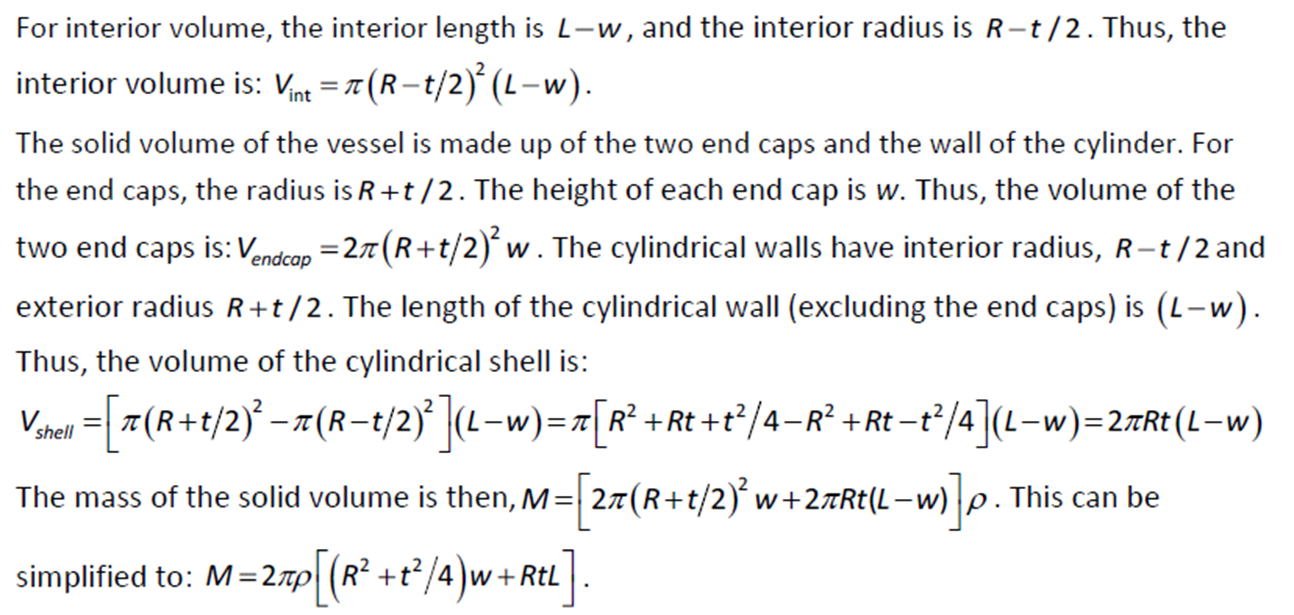
A closed-end, thin-walled cylindrical pressure vessel is shown in the figure below, the design objective is to select the mean radius R and wall thickness t to minimize the total mass. The vessel should contain at least 40.0 m of gas at an internal pressure of P = 6.0 MPa. It is required that the circumferential stress in the pressure vessel not exceed 250 MPa and the circumferential strain not exceed 2.0103. The circumferential stress and strain are calculated from the equations: PR PR(2-v) 2Et where is the circumferential stress (in Pa) and is the circumferential strain. Parameters describing the vessel are: p = density of vessel E = Young's modulus =8000 kg/m =200 GPa v = Poisson's ratio L= Length of vessel =0.30 =10.0 m w= Thickness of endcap = 5.0 cm Gas L = 10.0 m 5.0 cm PR For interior volume, the interior length is L-w, and the interior radius is R-t/2. Thus, the interior volume is: Vint =(R-t/2) (L-w). The solid volume of the vessel is made up of the two end caps and the wall of the cylinder. For the end caps, the radius is R+t/2. The height of each end cap is w. Thus, the volume of the two end caps is: Vendcap =2 (R+t/2) w. The cylindrical walls have interior radius, R-t/2 and exterior radius R+t/2. The length of the cylindrical wall (excluding the end caps) is (L-w). Thus, the volume of the cylindrical shell is: V shell = [ (R+t/2) (R t/2)](Lw)=[R +Rt+t/4-R+Rtt/4](L-w)=2Rt (Lw) The mass of the solid volume is then, M = [2(R+t/2)w+2Rt(Lw)]p. This can be simplified to: M=2p[(R +t/4)w+RtL].