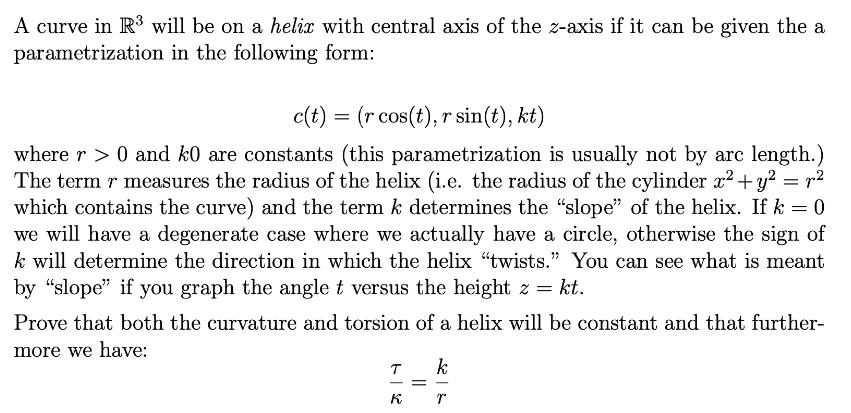
A curve in R will be on a helix with central axis of the z-axis if it can be given the a parametrization in the following form: c(t) = (r cos(t), r sin(t), kt) where r> 0 and k0 are constants (this parametrization is usually not by arc length.) The term r measures the radius of the helix (i.e. the radius of the cylinder x + y = r which contains the curve) and the term k determines the "slope" of the helix. If k = 0 we will have a degenerate case where we actually have a circle, otherwise the sign of k will determine the direction in which the helix "twists." You can see what is meant by "slope" if you graph the angle t versus the height z = kt. Prove that both the curvature and torsion of a helix will be constant and that further- more we have: T K = k T