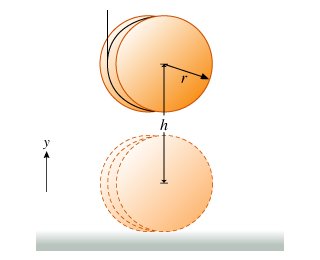
A cylinder with a moment of inertia I (about its axis of symmetry), mass m, and radius r has a massless string wrapped around it which is tied to the ceiling (Figure 1).

At time t=0 the cylinder is released from rest at height h above the ground. Use g for the magnitude of the acceleration of gravity. Assume that the string does not slip on the cylinder. Let v represent the instantaneous velocity of the center of mass of the cylinder, and let ? represent the instantaneous angular velocity of the cylinder about its center of mass. Note that there are no horizontal forces present, so for this problem v=?vj^ and ?=??k^.

A. The string constrains the rotational and translational motion of the falling cylinder, given that it doesn't slip. What is the relationship between the magnitude of the angular velocity ? and that of the velocity v of the center of mass of the cylinder? Express ? in terms of v and r.

B. Now return to the original cylinder. Using Newton's 2nd law, complete the equation of motion in the vertical direction j^ that describes the translational motion of the cylinder. Express your answer in terms of the tension T in the vertical section of string, m, and g; a positive answer indicates an upward acceleration.

C. Using the equation of rotational motion and the definition of torque ? = r * F, complete the equation of rotational motion of the cylinder about its center of mass. Your answer should include the tension T in the vertical section of string and the radius r. A positive answer indicates a counterclockwise torque about the center of mass (in the k^ unit direction).

D. In other parts of this problem expressions have been found for the vertical acceleration of the cylinder a_y and the angular acceleration ? of the cylinder in the k^ direction; both expressions include an unknown variable, namely, the tension T in the vertical section of string. The string constrains the rotational and vertical motions, providing a third equation relating a_y and ?. Solve these three equations to find the vertical acceleration, a_y, of the center of mass of the cylinder. Express a_y in terms of g, m, r, and I; a positive answer indicates upward acceleration.

E. Solve for the tension T in the vertical section of string. Express T in terms of the known variables I, m, r, and g.