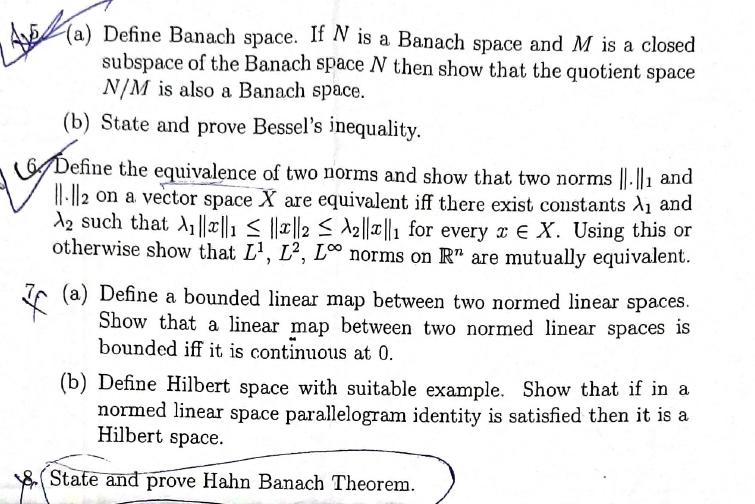
(a) Define Banach space. If N is a Banach space and M is a closed subspace of the Banach space N then show that the quotient space N/M is also a Banach space. (b) State and prove Bessel's inequality. Define the equivalence of two norms and show that two norms ||-||1 and 2 on a vector space X are equivalent iff there exist constants A and A2 such that A||x1222||1 for every x E X. Using this or otherwise show that L1, L2, L norms on R" are mutually equivalent. (a) Define a bounded linear map between two normed linear spaces. Show that a linear map between two normed linear spaces is bounded iff it is continuous at 0. (b) Define Hilbert space with suitable example. Show that if in a normed linear space parallelogram identity is satisfied then it is a Hilbert space. (State and prove Hahn Banach Theorem.