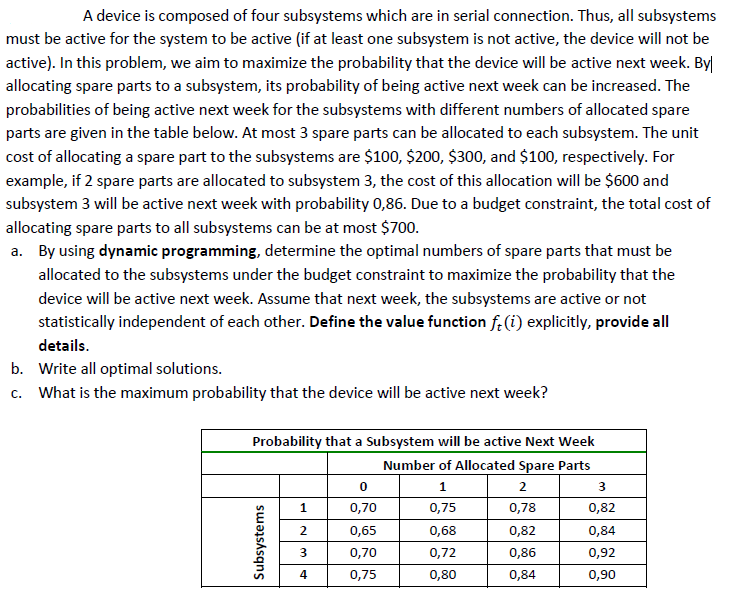
A device is composed of four subsystems which are in serial connection. Thus, all subsystems must be active for the system to be active (if at least one subsystem is not active, the device will not be active). In this problem, we aim to maximize the probability that the device will be active next week. Byl allocating spare parts to a subsystem, its probability of being active next week can be increased. The probabilities of being active next week for the subsystems with different numbers of allocated spare parts are given in the table below. At most 3 spare parts can be allocated to each subsystem. The unit cost of allocating a spare part to the subsystems are $100, $200, $300, and $100, respectively. For example, if 2 spare parts are allocated to subsystem 3, the cost of this allocation will be $600 and subsystem 3 will be active next week with probability 0,86. Due to a budget constraint, the total cost of allocating spare parts to all subsystems can be at most $700. a. By using dynamic programming, determine the optimal numbers of spare parts that must be allocated to the subsystems under the budget constraint to maximize the probability that the device will be active next week. Assume that next week, the subsystems are active or not statistically independent of each other. Define the value function f: (i) explicitly, provide all details. b. Write all optimal solutions. C. What is the maximum probability that the device will be active next week? Subsystems Probability that a Subsystem will be active Next Week Number of Allocated Spare Parts 0 1 2 3 1 0,70 0,75 0,78 0,82 2 0,65 0,68 0,82 0,84 3 0,70 0,72 0,86 0,92 4 0,75 0,80 0,84 0,90 A device is composed of four subsystems which are in serial connection. Thus, all subsystems must be active for the system to be active (if at least one subsystem is not active, the device will not be active). In this problem, we aim to maximize the probability that the device will be active next week. Byl allocating spare parts to a subsystem, its probability of being active next week can be increased. The probabilities of being active next week for the subsystems with different numbers of allocated spare parts are given in the table below. At most 3 spare parts can be allocated to each subsystem. The unit cost of allocating a spare part to the subsystems are $100, $200, $300, and $100, respectively. For example, if 2 spare parts are allocated to subsystem 3, the cost of this allocation will be $600 and subsystem 3 will be active next week with probability 0,86. Due to a budget constraint, the total cost of allocating spare parts to all subsystems can be at most $700. a. By using dynamic programming, determine the optimal numbers of spare parts that must be allocated to the subsystems under the budget constraint to maximize the probability that the device will be active next week. Assume that next week, the subsystems are active or not statistically independent of each other. Define the value function f: (i) explicitly, provide all details. b. Write all optimal solutions. C. What is the maximum probability that the device will be active next week? Subsystems Probability that a Subsystem will be active Next Week Number of Allocated Spare Parts 0 1 2 3 1 0,70 0,75 0,78 0,82 2 0,65 0,68 0,82 0,84 3 0,70 0,72 0,86 0,92 4 0,75 0,80 0,84 0,90