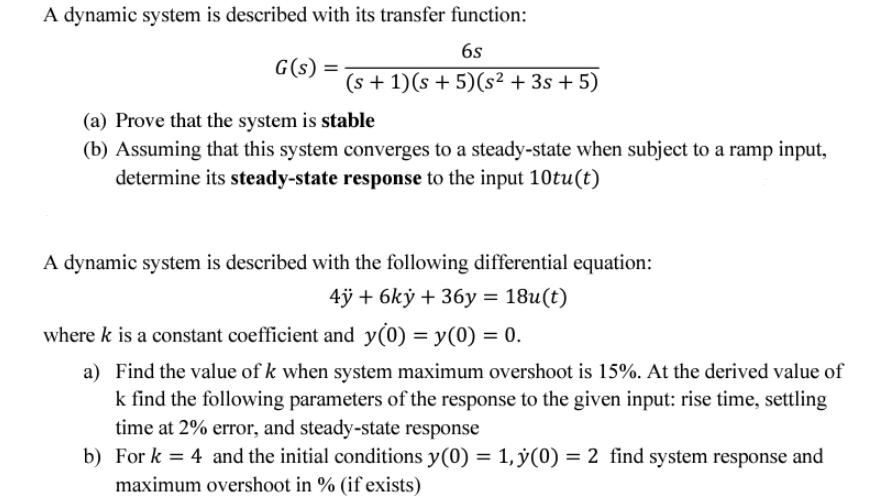
A dynamic system is described with its transfer function: 6s (s + 1)(s + 5) (s + 3s + 5) G(s) (a) Prove that the system is stable (b) Assuming that this system converges to a steady-state when subject to a ramp input, determine its steady-state response to the input 10tu(t) A dynamic system is described with the following differential equation: 4 + 6k + 36y = 18u(t) where k is a constant coefficient and y(0) = y(0) = 0. a) Find the value of k when system maximum overshoot is 15%. At the derived value of k find the following parameters of the response to the given input: rise time, settling time at 2% error, and steady-state response b) For k= 4 and the initial conditions y(0) = 1, y(0) = 2 find system response and maximum overshoot in % (if exists)