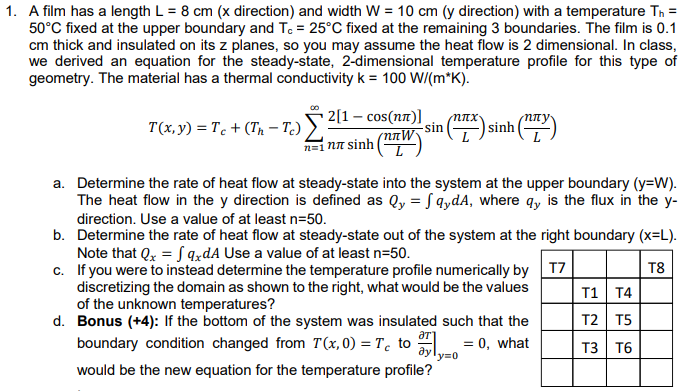
A film has a length L=8cm ( x direction) and width W=10cm ( y direction) with a temperature Th= 50C fixed at the upper boundary and Tc=25C fixed at the remaining 3 boundaries. The film is 0.1 cm thick and insulated on its z planes, so you may assume the heat flow is 2 dimensional. In class, we derived an equation for the steady-state, 2-dimensional temperature profile for this type of geometry. The material has a thermal conductivity k=100W/(mK). T(x,y)=Tc+(ThTc)n=1nsinh(LnW)2[1cos(n)]sin(Lnx)sinh(Lny) a. Determine the rate of heat flow at steady-state into the system at the upper boundary (y=W). The heat flow in the y direction is defined as Qy=qydA, where qy is the flux in the y direction. Use a value of at least n=50. b. Determine the rate of heat flow at steady-state out of the system at the right boundary (x=L). Note that Qx=qxdA Use a value of at least n=50. c. If you were to instead determine the temperature profile numerically by discretizing the domain as shown to the right, what would be the values of the unknown temperatures? d. Bonus (+4): If the bottom of the system was insulated such that the boundary condition changed from T(x,0)=Tc to yTy=0=0, what would be the new equation for the temperature profile? A film has a length L=8cm ( x direction) and width W=10cm ( y direction) with a temperature Th= 50C fixed at the upper boundary and Tc=25C fixed at the remaining 3 boundaries. The film is 0.1 cm thick and insulated on its z planes, so you may assume the heat flow is 2 dimensional. In class, we derived an equation for the steady-state, 2-dimensional temperature profile for this type of geometry. The material has a thermal conductivity k=100W/(mK). T(x,y)=Tc+(ThTc)n=1nsinh(LnW)2[1cos(n)]sin(Lnx)sinh(Lny) a. Determine the rate of heat flow at steady-state into the system at the upper boundary (y=W). The heat flow in the y direction is defined as Qy=qydA, where qy is the flux in the y direction. Use a value of at least n=50. b. Determine the rate of heat flow at steady-state out of the system at the right boundary (x=L). Note that Qx=qxdA Use a value of at least n=50. c. If you were to instead determine the temperature profile numerically by discretizing the domain as shown to the right, what would be the values of the unknown temperatures? d. Bonus (+4): If the bottom of the system was insulated such that the boundary condition changed from T(x,0)=Tc to yTy=0=0, what would be the new equation for the temperature profile