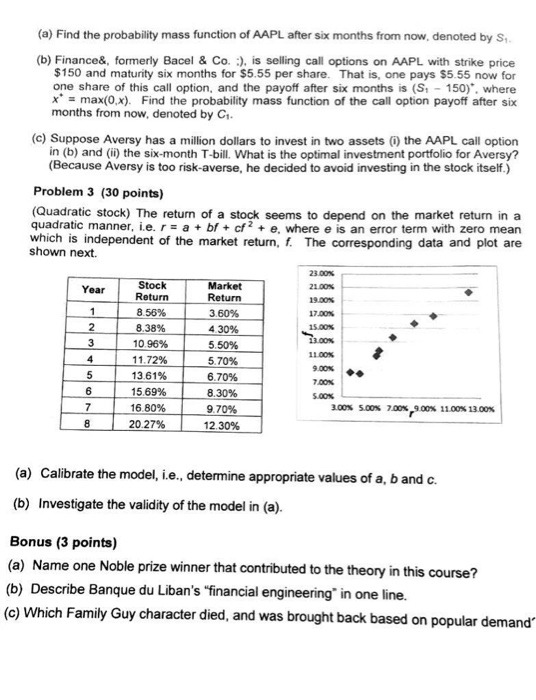
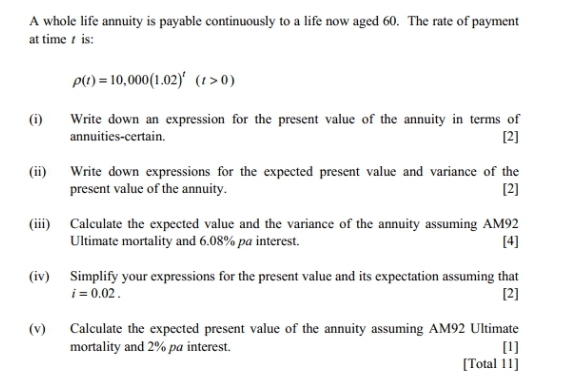

(a) Find the probability mass function of AAPL after six months from now, denoted by S,. (b) Finance&, formerly Bacel & Co. :), is selling call options on AAPL with strike price $150 and maturity six months for $5.55 per share. That is, one pays $5.55 now for one share of this call option, and the payoff after six months is (S1 - 150)", where x" = max(0,x). Find the probability mass function of the call option payoff after six months from now, denoted by C. (c) Suppose Aversy has a million dollars to invest in two assets (i) the AAPL call option in (b) and (ii) the six-month T-bill. What is the optimal investment portfolio for Aversy? (Because Aversy is too risk-averse, he decided to avoid investing in the stock itself.) Problem 3 (30 points) (Quadratic stock) The return of a stock seems to depend on the market return in a quadratic manner, i.e. r = a + b/ + of0) (i) Write down an expression for the present value of the annuity in terms of annuities-certain. [2] (1i) Write down expressions for the expected present value and variance of the present value of the annuity. [2] (iii) Calculate the expected value and the variance of the annuity assuming AM92 Ultimate mortality and 6.08% pa interest. [4] (iv) Simplify your expressions for the present value and its expectation assuming that i = 0.02. [2] (v) Calculate the expected present value of the annuity assuming AM92 Ultimate mortality and 2% pa interest. [1] [Total 11]A whole life annuity is payable continuously to a life now aged 60. The rate of payment at time / is: p() = 10,000(1.02)' (1 >0) (i) Write down an expression for the present value of the annuity in terms of annuities-certain. [2] (1i) Write down expressions for the expected present value and variance of the present value of the annuity. [2] (iii) Calculate the expected value and the variance of the annuity assuming AM92 Ultimate mortality and 6.08% pa interest. [4] (iv) Simplify your expressions for the present value and its expectation assuming that i = 0.02. [2] (v) Calculate the expected present value of the annuity assuming AM92 Ultimate mortality and 2% pa interest. [1] [Total 11]Question 5 Please show all calculations to get credit (8 points) a. Penny purchases two goods, food (F) and clothing (C). The utility that Ann receives by consuming food (F) and clothing C is given by U(F, C) = FC. She has income / = 240 and faces prices P. = $8 and Py = $2The Marginal Rate of Substitution (MRS) is - and the equation of the budget line is given by I = PFF + PCC. The tangency condition requires that MRS is equal to the slope of the budget line - -5. Write the Statement of the problem, Lagrangian, First Order Conditions as done in class and find out the equilibrium quantity of purchase of F* and C*. This is the baseline case. Now, If the price of clothing increases to $8 (everything else remaining unchanged) rewrite the Statement of the problem, Lagrangian, First Order Conditions as done in class and find out the new equilibrium quantity of purchase of F and C; call them F*new and C'new. This is called Case 1. (2.5 points + 2.5 points =5 points) b. What must the price of x fall to in order for her to be exactly as well off as before the change in Py i.e. We need to calculate px such that, with the new prices of product y, Penny reaches exactly the same indifference curve as in the baseline case? (3 points) Formula Sheet MU, = 204; for one variable; MU, = ducey). dx ax : MUy = -for two variables Max(xyU = U(x, y); I = Pxx + Pyy ; L = U(x, y) + A(I -PxX - Py>) MUx = Px Tangency Condition: MU, Py MPL = -90(L.K). A al. ; MPK = -30(L,K) ak Min[LK] TC = WL + rK; Q = Q(L, K) ; [ = WL + rk + A(Q - Q(L.K)) . MPL - W Tangency Condition: MPK Steps to Utility Maximization Steps to Cost Minimization & minimized cost function Stop 1: Find the production function and set the quantity Identify the utility function target - this is your constraint (Isoquant] Identify the budget constraint Step 2: Find the (unminimized) cost function - this is your objective function (socoit) Write the choice function Step A: Write the statement of the problem: what do you Write the lagrangian want to minimize? Subject to what constraintr variables are endogenous? What variables are exogenous . Find out the 3 first order conditions Step 4: Find out the first order conditions & use them to . Use the first two first order conditions to find out bolate the tangency condition and the constraint the tangency condition Step S: Compute equilibrium employment of labor, and . Use the tangency condition and the third first capital as a function of the exogenous parameters Le. the factor demand functions order condition to find the optimal consumption Step 6: Stick in the factor demand functions into the cost of x and y and U function to find out the minimized cost function














