Answered step by step
Verified Expert Solution
Question
1 Approved Answer
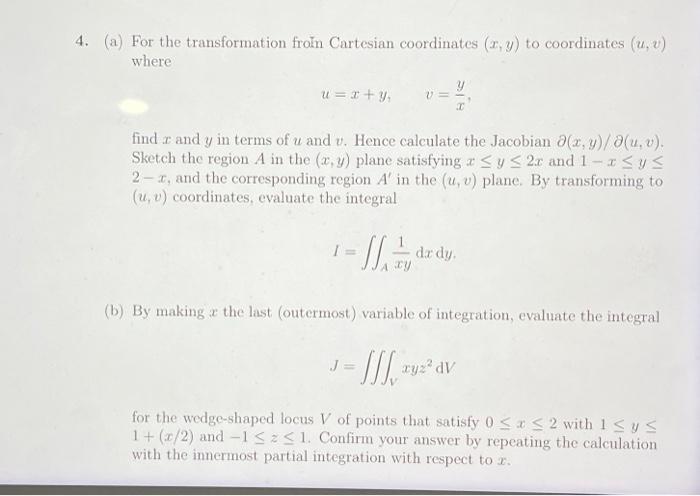
(a) For the transformation from Cartesian coordinates ( (x, y) ) to coordinates ( (u, v) ) where [ u=x+y, quad v=rac{y}{x} ] find (
(a) For the transformation from Cartesian coordinates ( (x, y) ) to coordinates ( (u, v) ) where [ u=x+y, quad v=rac{y}{x} ] find ( x ) and ( y ) in terms of ( u ) and ( v ). Hence calculate the Jacobian ( partial(x, y) / partial(u, v) ). Sketch the region ( A ) in the ( (x, y) ) plane satisfying ( x leq y leq 2 x ) and ( 1-x leq y leq ) ( 2-x ), and the corresponding region ( A^{prime} ) in the ( (u, v) ) plane. By transforming to ( (u, v) ) coordinates, evaluate the integral [ I=iint_{A} rac{1}{x y} mathrm{~d} x mathrm{~d} y ] (b) By making ( x ) the last (outermost) variable of integration, evaluate the integral [ J=iiint_{V} x y z^{2} mathrm{~d} V ] for the wedge-shaped locus ( V ) of points that satisfy ( 0 leq x leq 2 ) with ( 1 leq y leq ) ( 1+(x / 2) ) and ( -1 leq z leq 1 ). Confirm your answer by repeating the calculation with the innermost partial integration with respect to ( x ).
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


