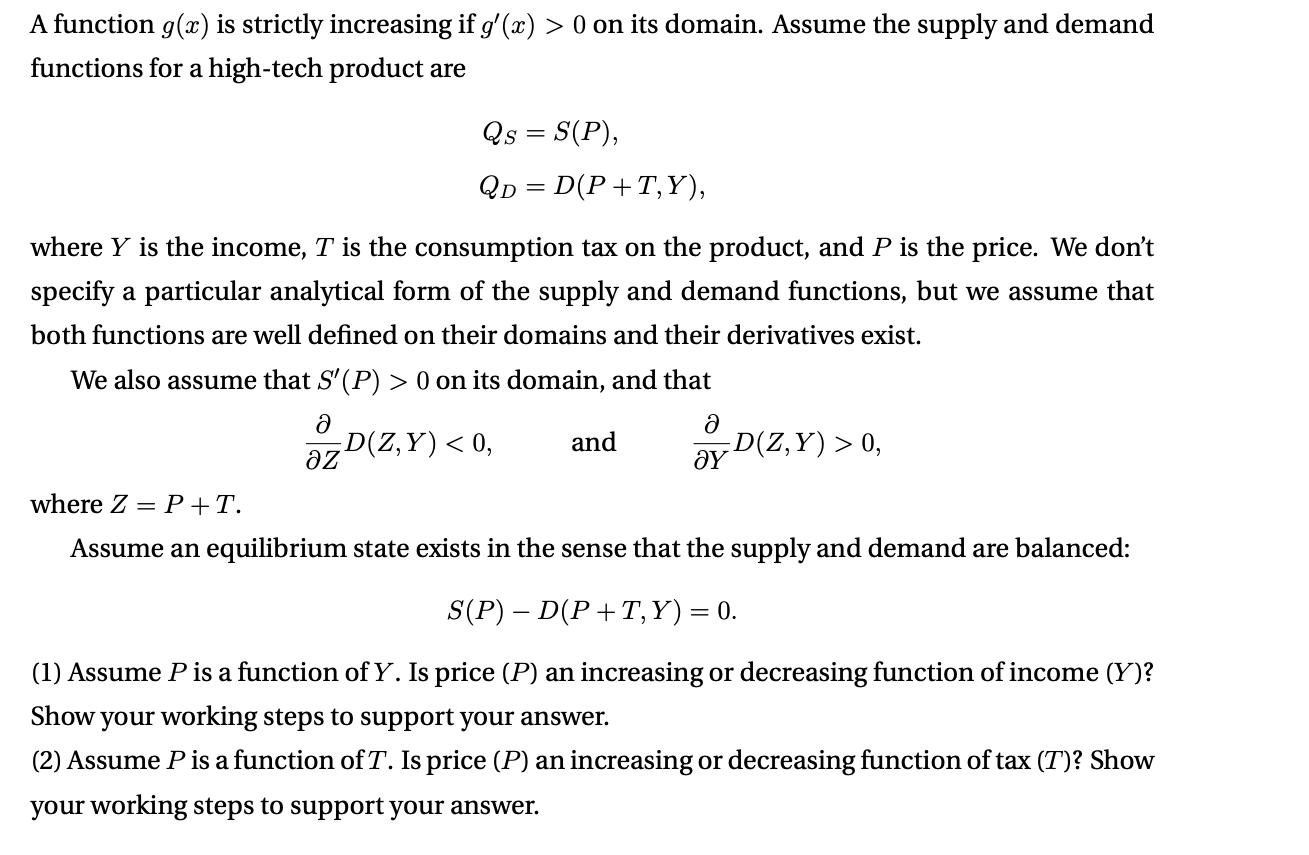
A function g(x) is strictly increasing if g'(x) > 0 on its domain. Assume the supply and demand functions for a high-tech product are Qs = S(P), QD = D(P+T, Y), where Y is the income, T is the consumption tax on the product, and P is the price. We don't specify a particular analytical form of the supply and demand functions, but we assume that both functions are well defined on their domains and their derivatives exist. We also assume that S'(P) > 0 on its domain, and that D(Z,Y) < 0, Y z and D(Z,Y) > 0, where Z P + T. Assume an equilibrium state exists in the sense that the supply and demand are balanced: S(P) - D(P+T, Y) = 0. (1) Assume P is a function of Y. Is price (P) an increasing or decreasing function of income (Y)? Show your working steps to support your answer. (2) Assume P is a function of T. Is price (P) an increasing or decreasing function of tax (T)? Show your working steps to support your answer.