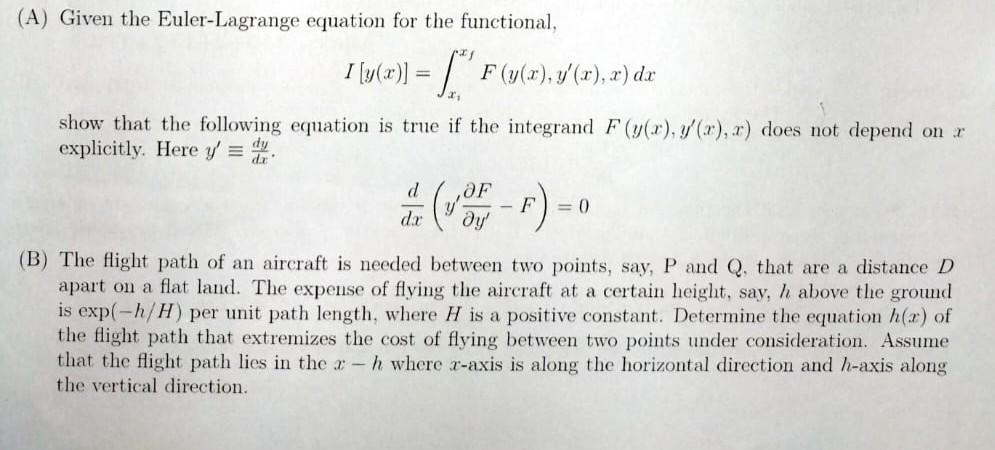
(A) Given the Euler-Lagrange equation for the functional, I[y(x)]=xixfF(y(x),y(x),x)dx show that the following equation is true if the integrand F(y(x),y(x),x) does not depend on x explicitly. Here ydxdy. dxd(yyFF)=0 (B) The flight path of an aircraft is needed between two points, say, P and Q, that are a distance D apart on a flat land. The expense of flying the aircraft at a certain height, say, h above the ground is exp(h/H) per unit path length, where H is a positive constant. Determine the equation h(x) of the flight path that extremizes the cost of flying between two points under consideration. Assume that the flight path lies in the xh where x-axis is along the horizontal direction and h-axis along the vertical direction