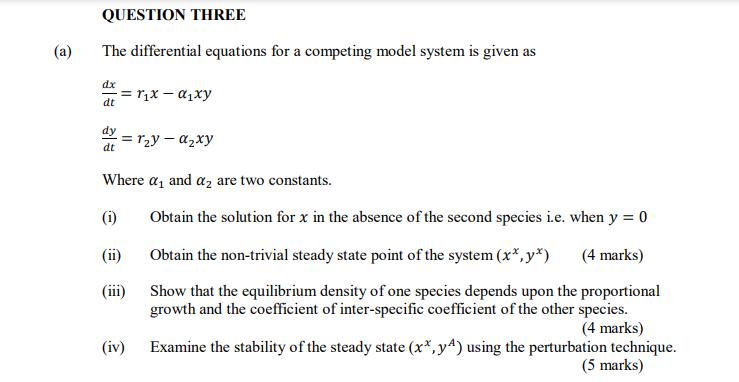
(a) QUESTION THREE The differential equations for a competing model system is given as dx dt dt =rx - xy = ry - xy Where and a are two constants. (1) Obtain the solution for x in the absence of the second species i.e. when y = 0 Obtain the non-trivial steady state point of the system (xx,y*) (4 marks) Show that the equilibrium density of one species depends upon the proportional growth and the coefficient of inter-specific coefficient of the other species. (4 marks) Examine the stability of the steady state (x,y4) using the perturbation technique. (5 marks) (ii) (iii) (iv)