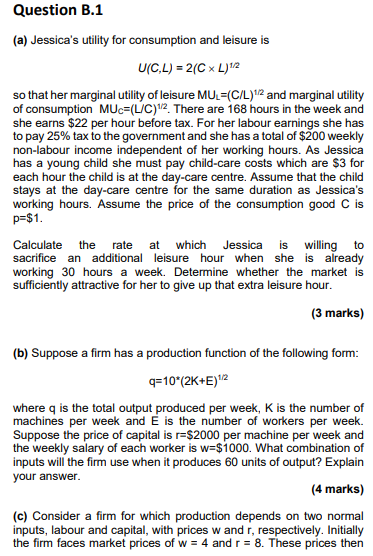

(a) Jessica's utility for consumption and leisure is U(C,L)=2(CL)1/2 so that her marginal utility of leisure MUL=(C/L)1/2 and marginal utility of consumption MUC=(L/C)1/2. There are 168 hours in the week and she earns $22 per hour before tax. For her labour earnings she has to pay 25% tax to the government and she has a total of $200 weekly non-labour income independent of her working hours. As Jessica has a young child she must pay child-care costs which are $3 for each hour the child is at the day-care centre. Assume that the child stays at the day-care centre for the same duration as Jessica's working hours. Assume the price of the consumption good C is p=$1. Calculate the rate at which Jessica is willing to sacrifice an additional leisure hour when she is already working 30 hours a week. Determine whether the market is sufficiently attractive for her to give up that extra leisure hour. (3 marks) (b) Suppose a firm has a production function of the following form: q=10(2K+E)1/2 where q is the total output produced per week, K is the number of machines per week and E is the number of workers per week. Suppose the price of capital is r=$2000 per machine per week and the weekly salary of each worker is w=$1000. What combination of inputs will the firm use when it produces 60 units of output? Explain your answer. (4 marks) (c) Consider a firm for which production depends on two normal inputs, labour and capital, with prices w and r, respectively. Initially the firm faces market prices of w=4 and r=8. These prices then shift to w=2 and r=4. What can you determine about the impacts of scale and substitution effects on capital and labour? (3 marks) (a) Jessica's utility for consumption and leisure is U(C,L)=2(CL)1/2 so that her marginal utility of leisure MUL=(C/L)1/2 and marginal utility of consumption MUC=(L/C)1/2. There are 168 hours in the week and she earns $22 per hour before tax. For her labour earnings she has to pay 25% tax to the government and she has a total of $200 weekly non-labour income independent of her working hours. As Jessica has a young child she must pay child-care costs which are $3 for each hour the child is at the day-care centre. Assume that the child stays at the day-care centre for the same duration as Jessica's working hours. Assume the price of the consumption good C is p=$1. Calculate the rate at which Jessica is willing to sacrifice an additional leisure hour when she is already working 30 hours a week. Determine whether the market is sufficiently attractive for her to give up that extra leisure hour. (3 marks) (b) Suppose a firm has a production function of the following form: q=10(2K+E)1/2 where q is the total output produced per week, K is the number of machines per week and E is the number of workers per week. Suppose the price of capital is r=$2000 per machine per week and the weekly salary of each worker is w=$1000. What combination of inputs will the firm use when it produces 60 units of output? Explain your answer. (4 marks) (c) Consider a firm for which production depends on two normal inputs, labour and capital, with prices w and r, respectively. Initially the firm faces market prices of w=4 and r=8. These prices then shift to w=2 and r=4. What can you determine about the impacts of scale and substitution effects on capital and labour








