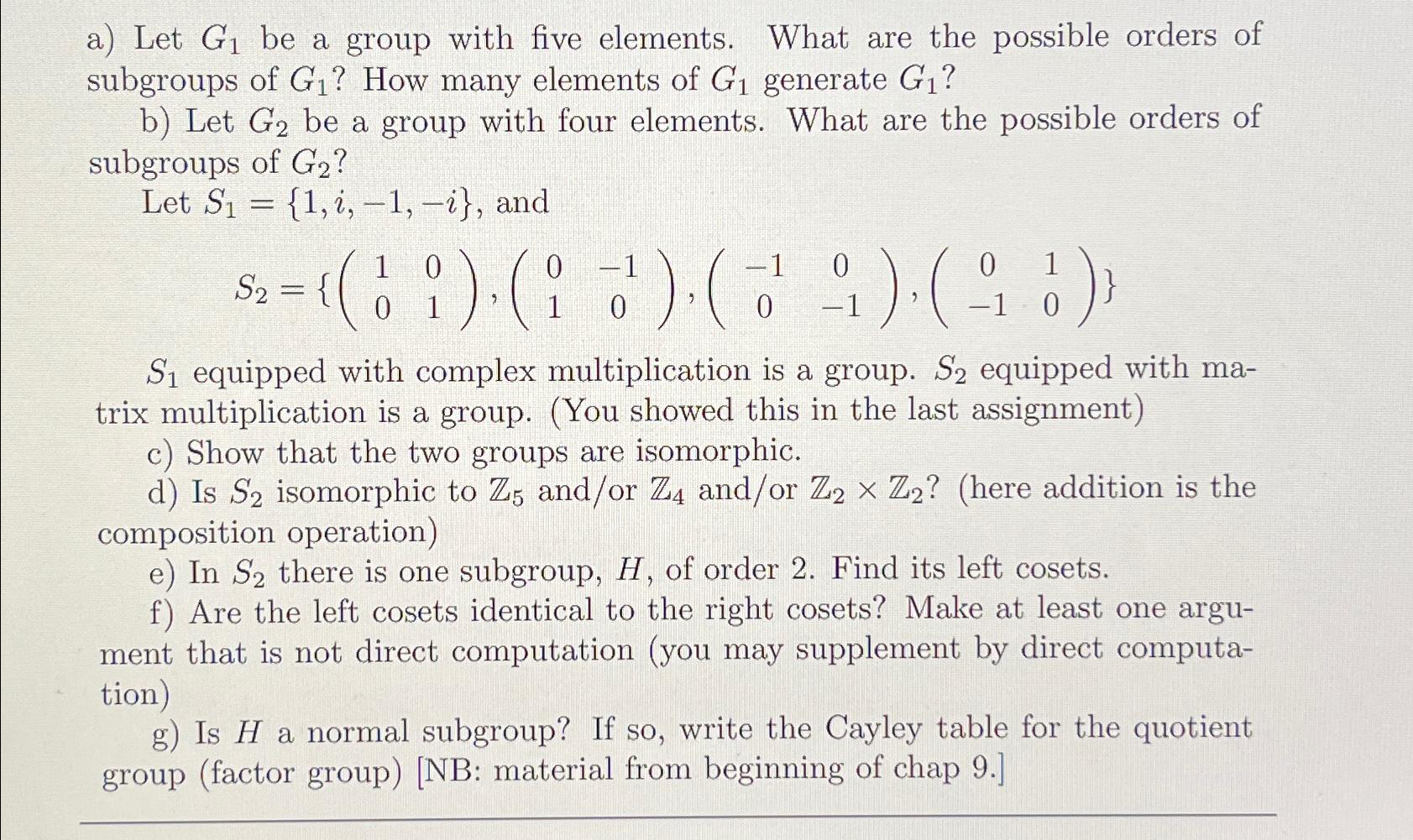
a) Let G be a group with five elements. What are the possible orders of subgroups of G? How many elements of G generate G? b) Let G2 be a group with four elements. What are the possible orders of subgroups of G? Let S = {1, i, -1, -1}, and S2 1 0 0 -1 0 0 -1 0 1 $ = i i 11 ; (( ). ( ) ( ) ( )) 0 -1 0 S equipped with complex multiplication is a group. S2 equipped with ma- trix multiplication is a group. (You showed this in the last assignment) c) Show that the two groups are isomorphic. d) Is S2 isomorphic to Z5 and/or Z4 and/or Z2 x Z2? (here addition is the composition operation) e) In S2 there is one subgroup, H, of order 2. Find its left cosets. f) Are the left cosets identical to the right cosets? Make at least one argu- ment that is not direct computation (you may supplement by direct computa- tion) g) Is H a normal subgroup? If so, write the Cayley table for the quotient group (factor group) [NB: material from beginning of chap 9.]