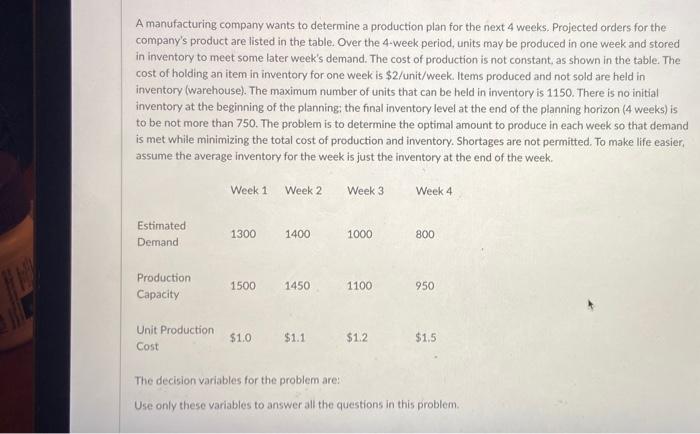
A manufacturing company wants to determine a production plan for the next 4 weeks. Projected orders for the company's product are listed in the table. Over the 4-week period, units may be produced in one week and stored in inventory to meet some later week's demand. The cost of production is not constant, as shown in the table. The cost of holding an item in inventory for one week is $2/unit/week. Items produced and not sold are held in inventory (warehouse). The maximum number of units that can be held in inventory is 1150. There is no initial inventory at the beginning of the planning the final inventory level at the end of the planning horizon (4 weeks) is to be not more than 750. The problem is to determine the optimal amount to produce in each week so that demand is met while minimizing the total cost of production and inventory Shortages are not permitted. To make life easier assume the average inventory for the week is just the inventory at the end of the week. Week 1 Week 2 Week 3 Week 4 Estimated Demand 1300 1400 1000 800 Production Capacity 1500 1450 1100 950 Unit Production Cost $1.0 $1.1 $1.2 $1,5 The decision variables for the problem are: Use only these variables to answer all the questions in this problem P1 = number of units to produce in week1 P2 = number of units to produce in week2 P3 - number of units to produce in week3 P4 = number of units to produce in week4 B1 -beginning inventory for week1 B2 -beginning inventory for week2 B3 =beginning inventory for week3 B4-beginning inventory for week4 B5 -beginning inventory for week5 Write the conservation constraints for production, inventory and demand ( this restrictions ensure that the beginning inventory in a given month equal the ending inventory from the previous month). Edit View Insert Format Tools Table 12ptParagraph IB I 09 al N Tvoor A manufacturing company wants to determine a production plan for the next 4 weeks. Projected orders for the company's product are listed in the table. Over the 4-week period, units may be produced in one week and stored in inventory to meet some later week's demand. The cost of production is not constant, as shown in the table. The cost of holding an item in inventory for one week is $2/unit/week. Items produced and not sold are held in inventory (warehouse). The maximum number of units that can be held in inventory is 1150. There is no initial inventory at the beginning of the planning the final inventory level at the end of the planning horizon (4 weeks) is to be not more than 750. The problem is to determine the optimal amount to produce in each week so that demand is met while minimizing the total cost of production and inventory Shortages are not permitted. To make life easier assume the average inventory for the week is just the inventory at the end of the week. Week 1 Week 2 Week 3 Week 4 Estimated Demand 1300 1400 1000 800 Production Capacity 1500 1450 1100 950 Unit Production Cost $1.0 $1.1 $1.2 $1,5 The decision variables for the problem are: Use only these variables to answer all the questions in this problem P1 = number of units to produce in week1 P2 = number of units to produce in week2 P3 - number of units to produce in week3 P4 = number of units to produce in week4 B1 -beginning inventory for week1 B2 -beginning inventory for week2 B3 =beginning inventory for week3 B4-beginning inventory for week4 B5 -beginning inventory for week5 Write the conservation constraints for production, inventory and demand ( this restrictions ensure that the beginning inventory in a given month equal the ending inventory from the previous month). Edit View Insert Format Tools Table 12ptParagraph IB I 09 al N Tvoor