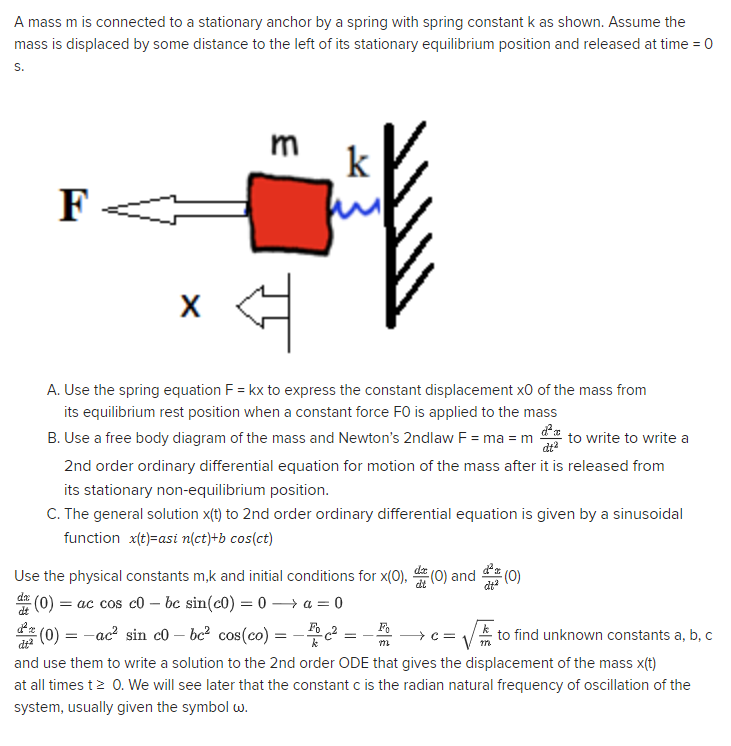
A mass m is connected to a stationary anchor by a spring with spring constant k as shown. Assume the mass is displaced by some distance to the left of its stationary equilibrium position and released at time = 0 S. F X (0) 3 A. Use the spring equation F = kx to express the constant displacement xO of the mass from its equilibrium rest position when a constant force FO is applied to the mass B. Use a free body diagram of the mass and Newton's 2ndlaw F = ma = m to write to write a 2nd order ordinary differential equation for motion of the mass after it is released from its stationary non-equilibrium position. C. The general solution x(t) to 2nd order ordinary differential equation is given by a sinusoidal function x(t)=asi n(ct)+b cos(ct) k Use the physical constants m,k and initial conditions for x(0), (0) and (0) a=0 = ac cos c0-bc sin(c0) = 0 (0) = -ac sin c0-bc cos(co) = / c =to find unknown constants a, b, c dt m and use them to write a solution to the 2nd order ODE that gives the displacement of the mass x(t) at all times t > 0. We will see later that the constant c is the radian natural frequency of oscillation of the system, usually given the symbol w. C=