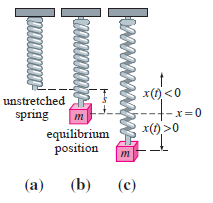
After a mass m is attached to a spring, it stretches it s units and then hangs
Question:
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

A First Course in Differential Equations with Modeling Applications
ISBN: 978-1305965720
11th edition
Authors: Dennis G. Zill
Question Posted:





