Answered step by step
Verified Expert Solution
Question
1 Approved Answer
a) Show that for a general M, i.e. not necessarily symmetric, the following equation is also satisfied (I) from (II) b) Since s^2 = 0
a) Show that for a general M, i.e. not necessarily symmetric, the following equation is also satisfied
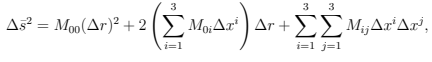 (I)
(I)
from 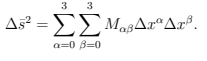 (II)
(II)
b) Since s^2 = 0 in (I) for any {x^i}, replace x^i by x^i in (I) and subtract the equation resulting from (I) to establish that M_0i = 0 for i = 1, 2, 3. c) Use (I) together with s^2 = 0 to set M_ij = (M_00)ij (i, j = 1, 2, 3).
Note: "_" is used to represent subscripts
3 3 3 g? = (r)2 + 2 (, *) r + ; ' , ** 2 (.., ) . 1=1 i=1 j=1 3 3 3 ? = :z3. 9 a=0 =0 3 3 3 g? = (r)2 + 2 (, *) r + ; ' , ** 2 (.., ) . 1=1 i=1 j=1 3 3 3 ? = :z3. 9 a=0 =0Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


