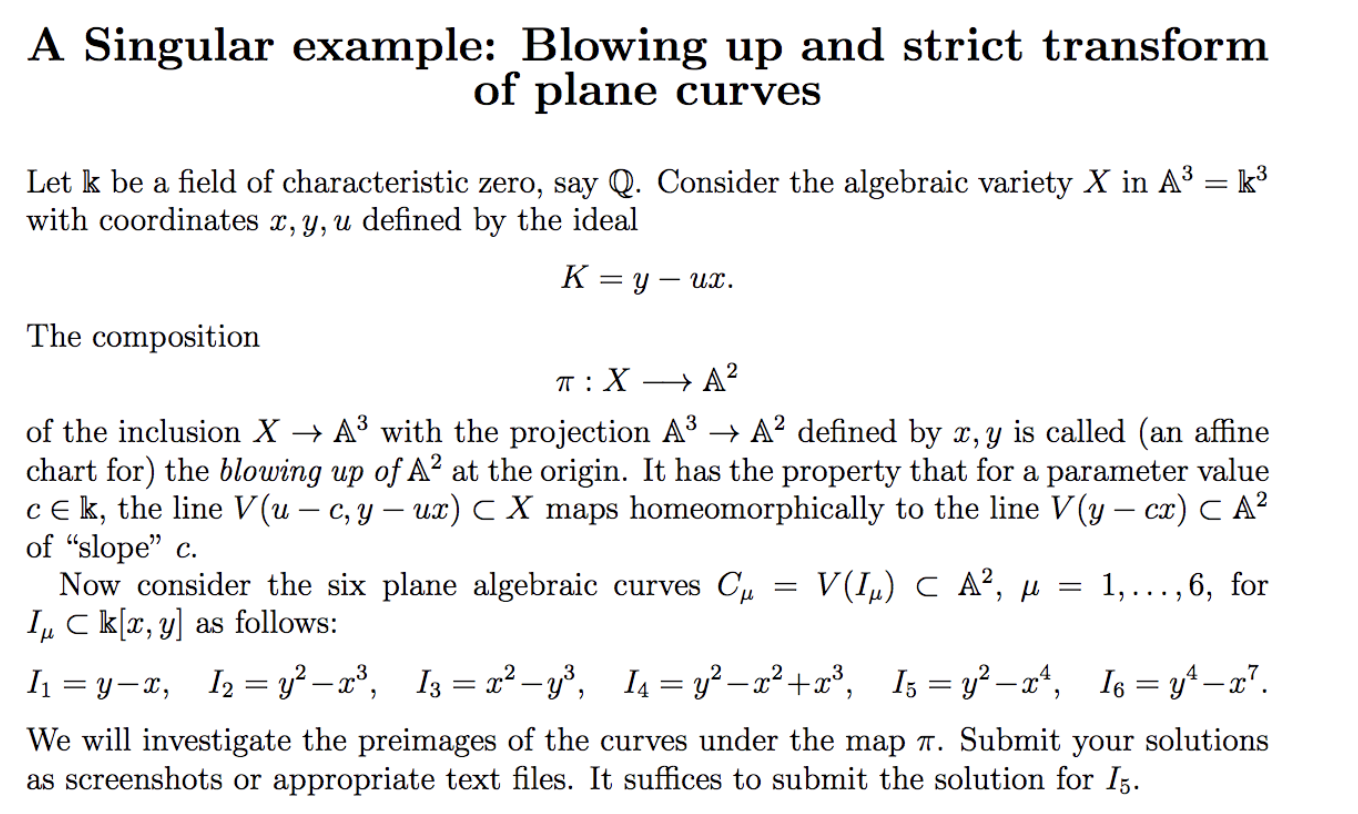
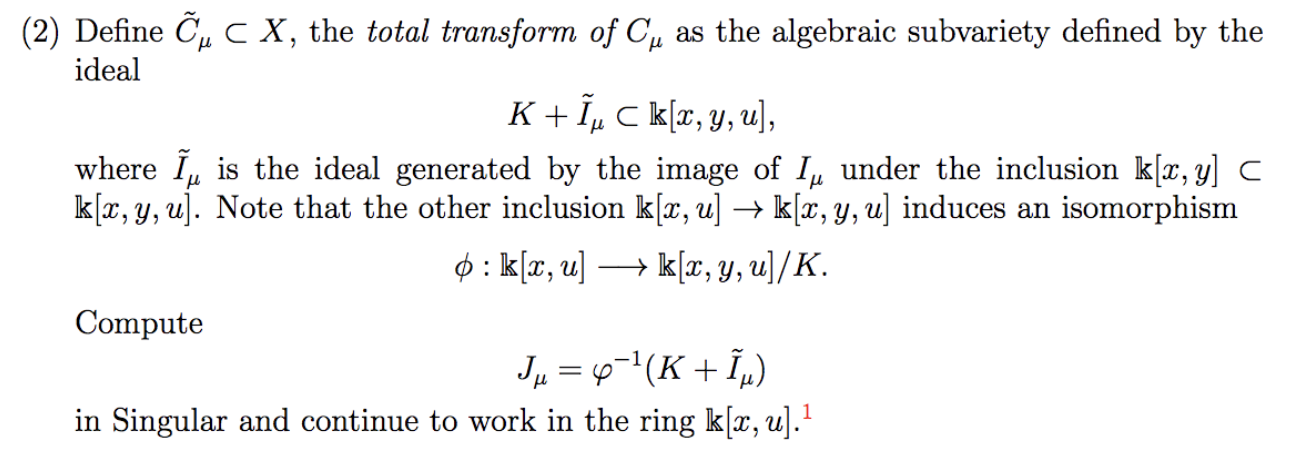
A Singular example: Blowing up and strict transform of plane curves Let k be a field of characteristic zero, say Q. Consider the algebraic variety X in A3 = k3 with coordinates x,y, u defined by the ideal K=y Ux. The composition T:X + A? of the inclusion X + A3 with the projection A3 + A defined by x,y is called (an affine chart for) the blowing up of A2 at the origin. It has the property that for a parameter value cek, the line V(u - C, Y ux) CX maps homeomorphically to the line V(y - cx) CA? of "slope c. Now consider the six plane algebraic curves Cu = V(Iu) C A, u = 1,...,6, for In C k[x, y) as follows: I1 = y-x, 12 = y2 2, I3 = x24), 14 = y2 x2+x?, 15 = y2 x4, 16 = y42?. We will investigate the preimages of the curves under the map 1. Submit your solutions as screenshots or appropriate text files. It suffices to submit the solution for 15. (2) Define M CX, the total transform of Cu as the algebraic subvariety defined by the ideal K +In C k[r, y, u], where I is the ideal generated by the image of In under the inclusion k[z, y] C k[x, y, u). Note that the other inclusion k[x, u] k[x, y, u) induces an isomorphism 0 : k[z, u] k[z, y, u]/K. Compute Ju = 4-(K + Iu) in Singular and continue to work in the ring k[x, ul.1 A Singular example: Blowing up and strict transform of plane curves Let k be a field of characteristic zero, say Q. Consider the algebraic variety X in A3 = k3 with coordinates x,y, u defined by the ideal K=y Ux. The composition T:X + A? of the inclusion X + A3 with the projection A3 + A defined by x,y is called (an affine chart for) the blowing up of A2 at the origin. It has the property that for a parameter value cek, the line V(u - C, Y ux) CX maps homeomorphically to the line V(y - cx) CA? of "slope c. Now consider the six plane algebraic curves Cu = V(Iu) C A, u = 1,...,6, for In C k[x, y) as follows: I1 = y-x, 12 = y2 2, I3 = x24), 14 = y2 x2+x?, 15 = y2 x4, 16 = y42?. We will investigate the preimages of the curves under the map 1. Submit your solutions as screenshots or appropriate text files. It suffices to submit the solution for 15. (2) Define M CX, the total transform of Cu as the algebraic subvariety defined by the ideal K +In C k[r, y, u], where I is the ideal generated by the image of In under the inclusion k[z, y] C k[x, y, u). Note that the other inclusion k[x, u] k[x, y, u) induces an isomorphism 0 : k[z, u] k[z, y, u]/K. Compute Ju = 4-(K + Iu) in Singular and continue to work in the ring k[x, ul.1