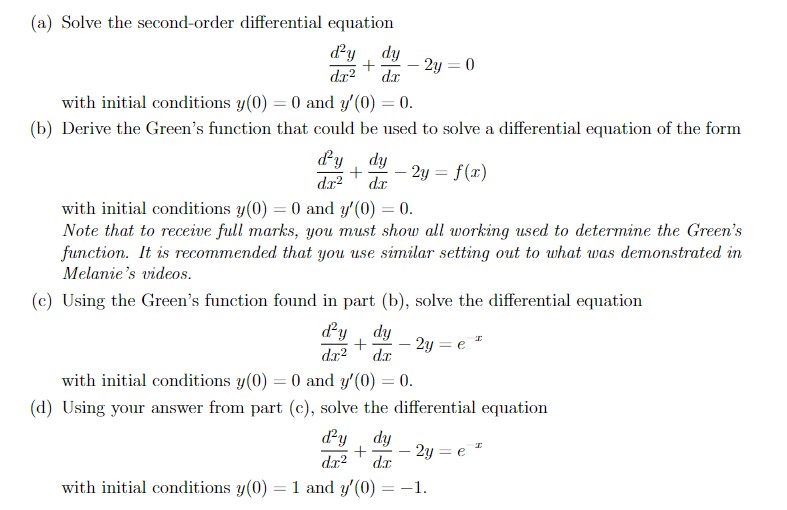
(a) Solve the second-order differential equation d'y dy d.x dx + with initial conditions y(0) = 0 and y'(0) 0. (b) Derive the Green's function that could be used to solve a differential equation of the form = 2y = 0 d'y dy + dr dx with initial conditions y(0) = 0 and y'(0) = 0. Note that to receive full marks, you must show all working used to determine the Green's function. It is recommended that you use similar setting out to what was demonstrated in Melanie's videos. (c) Using the Green's function found in part (b), solve the differential equation 2y = e d'y dy + dr d.x - 2y = f(x) with initial conditions y(0) = 0 and y'(0) - 0. (d) Using your answer from part (c), solve the differential equation d'y dy dx dx with initial conditions y(0) = 1 and y'(0) = -1. + - 2y = e "