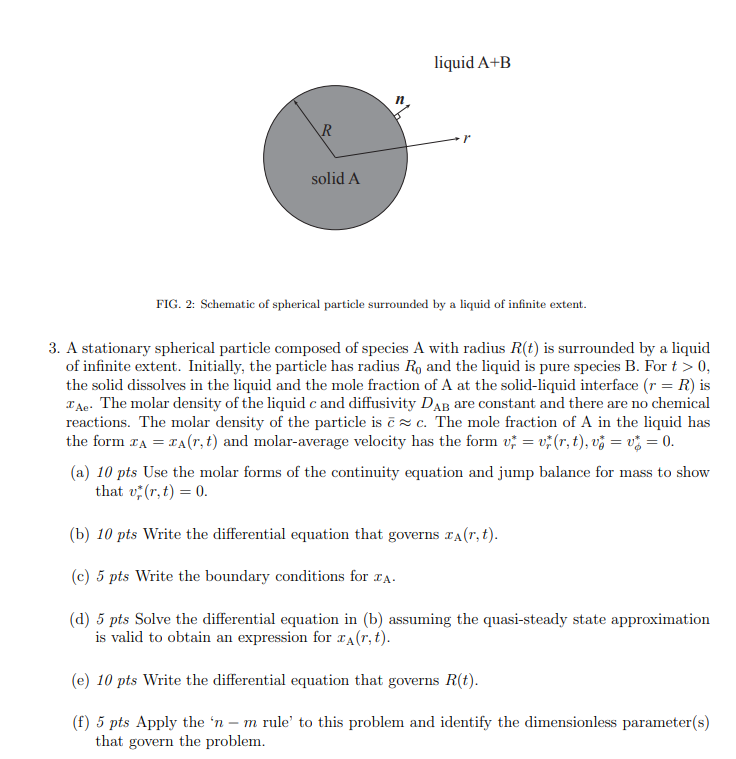
A stationary spherical particle composed of species A with radius $R(t)$ is surrounded by a liquid

of infinite extent. Initially, the particle has radius $R_0$ and the liquid is pure species $B.$ For $t>0,$

the solid dissolves in the liquid and the mole fraction of $A$ at the solid$-$liquid interface $(r=R)$ is

$x_{Ae}.$ The molar density of the liquid $c$ and diffusivity $D_{AB}$ are constant and there are no chemical

reactions. The molar density of the particle is $\frac{?}{\text{b}\text{a}\text{r}}(c)$~~$c.$ The mole fraction of $A$ in the liquid has

the form $x_A=x_A(r,t)$ and molar$-$average velocity has the form $v_r^{**}=v_r^{**}(r,t),v_{}^{**}=v_{}^{**}=0.$

$($a$)10$ pts Use the molar forms of the continuity equation and jump balance for mass to show

that $v_r^{**}(r,t)=0.$

$($b$)10pts$ Write the differential equation that governs $x_A(r,t).$

$($c$)5$ pts Write the boundary conditions for $x_A.$

$($d$)5$ pts Solve the differential equation in $($b$)$ assuming the quasi$-$steady state approximation

is valid to obtain an expression for $x_A(r,t).$

$($e$)10$ pts Write the differential equation that governs $R(t).$

$($f$)5$ pts Apply the $\text{'}n-m$ rule' to this problem and identify the dimensionless parameter$($s$)$

that govern the problem.