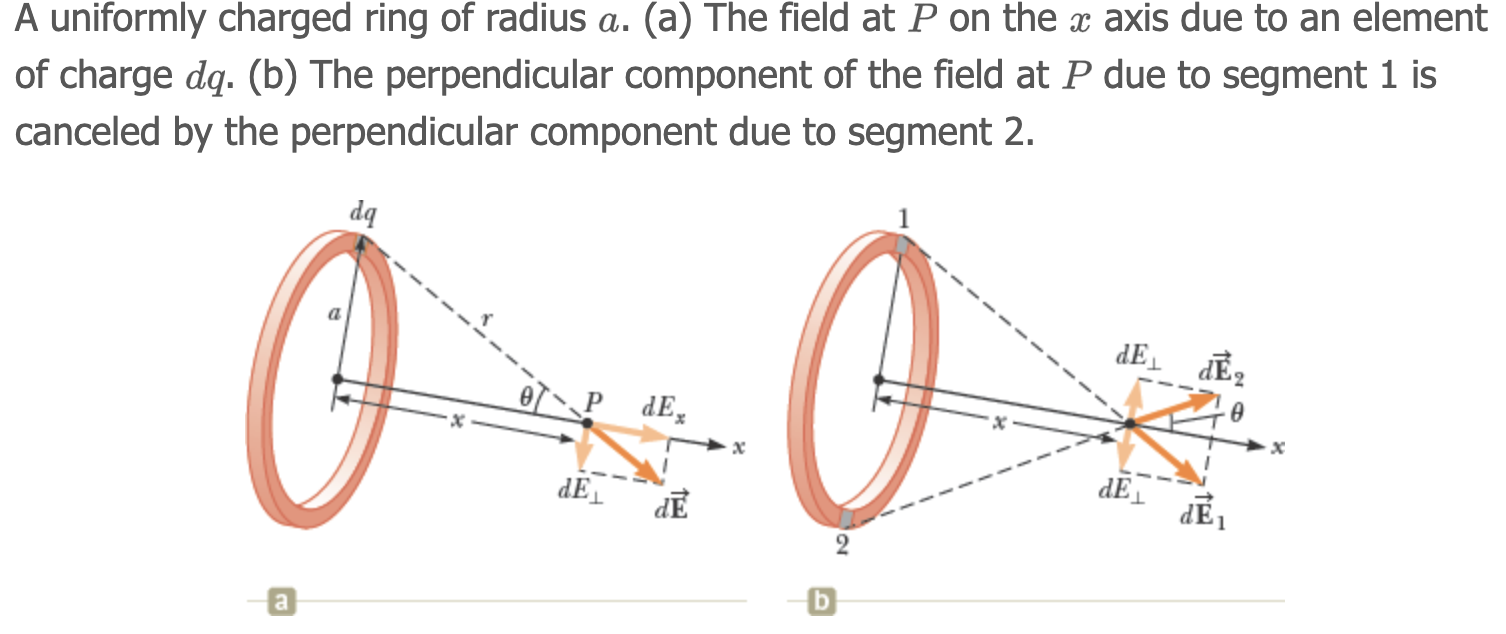
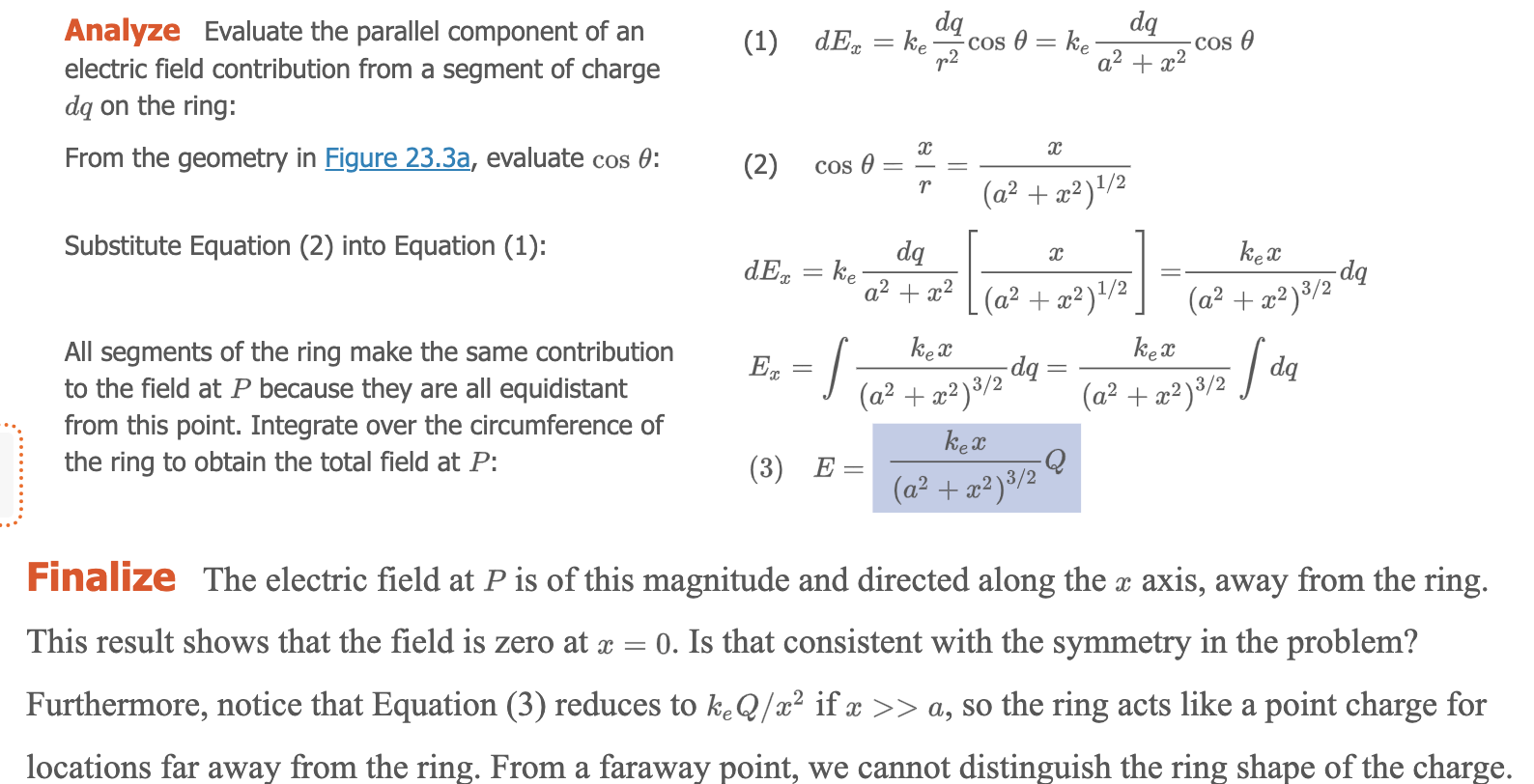
A uniformly charged ring of radius a. (a) The field at P on the x axis due to an element of charge dq. (b) The perpendicular component of the field at P due to segment 1 is canceled by the perpendicular component due to segment 2. dq OO dE dEx dE b 2 dE dE2 dE a Analyze Evaluate the parallel component of an electric field contribution from a segment of charge dq on the ring: From the geometry in Figure 23.3a, evaluate cos 0: Substitute Equation (2) into Equation (1): dq (1) dEx = ke x (2) cos A r dEx = ke All segments of the ring make the same contribution to the field at P because they are all equidistant from this point. Integrate over the circumference of the ring to obtain the total field at P: Ex cos 0 = ke dq a + x cos dq x (a + x) 1/2 a + x (a + x)1/2 kex (a + x)/2 kex kex = -dq = (a + -x2)/2 (a + x)/2 da kex (3) E = Q (a + x)/2 -dq Finalize The electric field at P is of this magnitude and directed along the x axis, away from the ring. This result shows that the field is zero at x = = 0. Is that consistent with the symmetry in the problem? Furthermore, notice that Equation (3) reduces to keQ/x if x >> a, so the ring acts like a point charge for locations far away from the ring. From a faraway point, we cannot distinguish the ring shape of the charge.