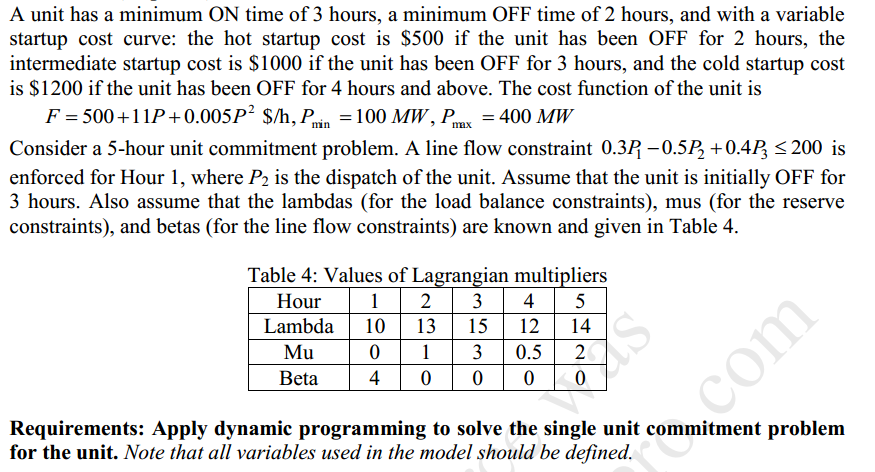
A unit has a minimum ON time of 3 hours, a minimum OFF time of 2 hours, and with a variable startup cost curve: the hot startup cost is $500 if the unit has been OFF for 2 hours, the intermediate startup cost is $1000 if the unit has been OFF for 3 hours, and the cold startup cost is $1200 if the unit has been OFF for 4 hours and above. The cost function of the unit is F 500+11P +0.005P2 S/h, P100 MW, P 400 MW Consider a 5-hour unit commitment problem. A line flow constraint 0.3R -0.5P +0.4R 3 200 is enforced for Hour 1, where P2 is the dispatch of the unit. Assume that the unit is initially OFF for 3 hours. Also assume that the lambdas (for the load balance constraints), mus (for the reserve constraints), and betas (for the line flow constraints) are known and given in Table 4 Table 4: Values of Lagrangian multipliers Hour 12345 Lambda10 13 15 1214 0 3 0.52 4 0 00 0 Beta Requirements: Apply dynamic programming to solve the single unit commitment problem for the unit. Note that all variables used in the model should be defined. A unit has a minimum ON time of 3 hours, a minimum OFF time of 2 hours, and with a variable startup cost curve: the hot startup cost is $500 if the unit has been OFF for 2 hours, the intermediate startup cost is $1000 if the unit has been OFF for 3 hours, and the cold startup cost is $1200 if the unit has been OFF for 4 hours and above. The cost function of the unit is F 500+11P +0.005P2 S/h, P100 MW, P 400 MW Consider a 5-hour unit commitment problem. A line flow constraint 0.3R -0.5P +0.4R 3 200 is enforced for Hour 1, where P2 is the dispatch of the unit. Assume that the unit is initially OFF for 3 hours. Also assume that the lambdas (for the load balance constraints), mus (for the reserve constraints), and betas (for the line flow constraints) are known and given in Table 4 Table 4: Values of Lagrangian multipliers Hour 12345 Lambda10 13 15 1214 0 3 0.52 4 0 00 0 Beta Requirements: Apply dynamic programming to solve the single unit commitment problem for the unit. Note that all variables used in the model should be defined