Question
a) Using the formal assumptions from the lecture and the reduction technique, prove that factoring is at least as hard as breaking the RSA assumption.
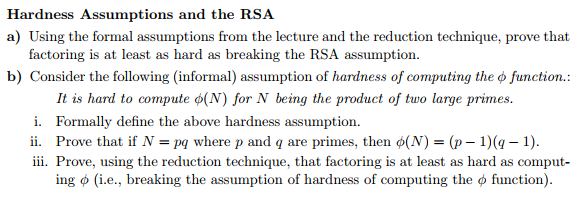
a) Using the formal assumptions from the lecture and the reduction technique, prove that factoring is at least as hard as breaking the RSA assumption. b) Consider the following (informal) assumption of hardness of computing the function.: It is hard to compute (N) for N being the product of two large primes. i. Formally define the above hardness assumption. ii. Prove that if N = pq where p and q are primes, then (N) = (p 1)(q 1). iii. Prove, using the reduction technique, that factoring is at least as hard as computing (i.e., breaking the assumption of hardness of computing the function).
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


