A water turbine is to be designed to produce 27 MW when running at 93.7 rev/min under a head

of 16.5 m. A model turbine with an output of 37.5 kW is to be tested under dynamically similar

conditions with a head of 4.9 m. Calculate the model speed and scale ratio. Assuming a model

efficiency of 88%, estimate the volume flow rate through the model. It is estimated that the force

on the thrust bearing of the full-size machine will be 7.0 GN. For what thrust must the model

bearing be designed?

5. Derive the non-dimensional groups that are normally used in the testing of gas turbines and

compressors. A compressor has been designed for normal atmospheric conditions (101.3 kPa

and 15C). To economise on the power required it is being tested with a throttle in the entry

duct to reduce the entry pressure. The characteristic curve for its normal design speed of 4000

rev/min is being obtained on a day when the ambient temperature is 20C. At what speed should

the compressor be run? At the point on the characteristic curve at which the mass flow would

normally be 58 kg/s the entry pressure is 55 kPa. Calculate the actual rate of mass flow during

the test.

6. Describe, with the aid of sketches, the relationship between geometry and specific speed for

pumps.

a. A model centrifugal pump with an impeller diameter of 20 cm is designed to rotate at 1450

rpm and to deliver 20 dm3

/s of fresh water against a pressure of 150 kPa. Determine the specific speed and diameter of the pump. How much power is needed to drive the pump if its

efficiency is 82%?

b. A prototype pump with an impeller diameter of 0.8 m is to be tested at 725 rpm under dynamically similar conditions as the model. Determine the head of water the pump must overcome, the volume flow rate, and the power needed to drive.

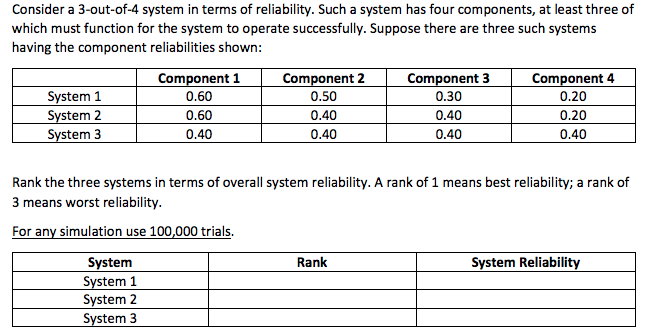
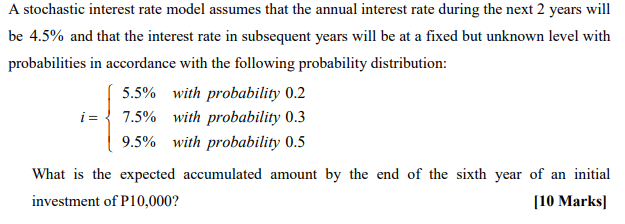
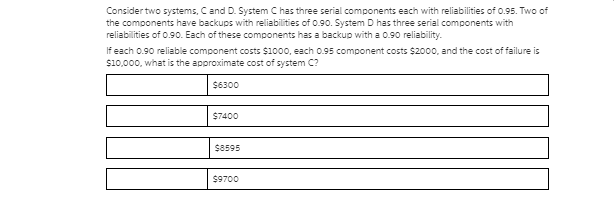
Data is collected on the time between arrivals of consecutive taxis at

a downtown hotel. We collect a data set of size 45 with sample mean x = 5.0 and sample

standard deviation s = 4.0.

(a) Assume the data follows a normal random variable.

(i) Find an 80% confidence interval for the mean of X.

(ii) Find an 80% ?

2

-confidence interval for the variance?

(b) Now make no assumptions about the distribution of of the data. By bootstrapping,

we generate 500 values for the differences ?

? = x

? ? x. The smallest and largest 150 are

written in non-decreasing order on the next page.

Use this data to find an 80% bootstrap confidence interval for .

(c) We suspect that the time between taxis is modeled by an exponential distribution, not

a normal distribution. In this case, are the approaches in the earlier parts justified?

(d) When might method (b) be preferable to method

Consider a 3-out-of-4 system in terms of reliability. Such a system has four components, at least three of which must function for the system to operate successfully. Suppose there are three such systems having the component reliabilities shown: Component 1 Component 2 Component 3 Component 4 System 1 0.60 0.50 0.30 0.20 System 2 0.60 0.40 0.40 0.20 System 3 0.40 0.40 0.40 0.40 Rank the three systems in terms of overall system reliability. A rank of 1 means best reliability; a rank of 3 means worst reliability. For any simulation use 100,000 trials. System Rank System Reliability System 1 System 2 System 3A stochastic interest rate model assumes that the annual interest rate during the next 2 years will be 4.5% and that the interest rate in subsequent years will be at a fixed but unknown level with probabilities in accordance with the following probability distribution: 5.5% with probability 0.2 7.5% with probability 0.3 9.5% with probability 0.5 What is the expected accumulated amount by the end of the sixth year of an initial investment of P10,000? [10 Marks]Statistics Quiz 2 Name: Date: A country conducts a study of new cars within the first 90 days of use. The cars have been categorized according to whether the car needs a warranty-based repair (yes or no) and the car's origin (domestic or foreign). Based on the data collected, the probability that the new car needs warranty repair is 0.09, the probability that the car was manufactured by a domestic company is 0.73, and the probability that the new car needs a warranty repair and was manufactured by a domestic company is 0.029. Complete the contingency table found below to evaluate the probabilities of a warranty-related repair. Yes (Y) No (Y') Domestic(D) Foreign (D') 1. What is the probability that a new car selected at random needs a warranty repair? 2. What is the probability that a new car selected at random does not need a warranty repair? 3. What is the probability that a new car selected at random is foreign manufactured? 4. What is the probability that a new car selected at random is domestically manufactured? 5. What is the probability that a new car selected at random needs a warranty repair and is domestically manufactured? 6. What is the probability that a new car selected at random needs a warranty repair or was manufactured by a domestic company?\f