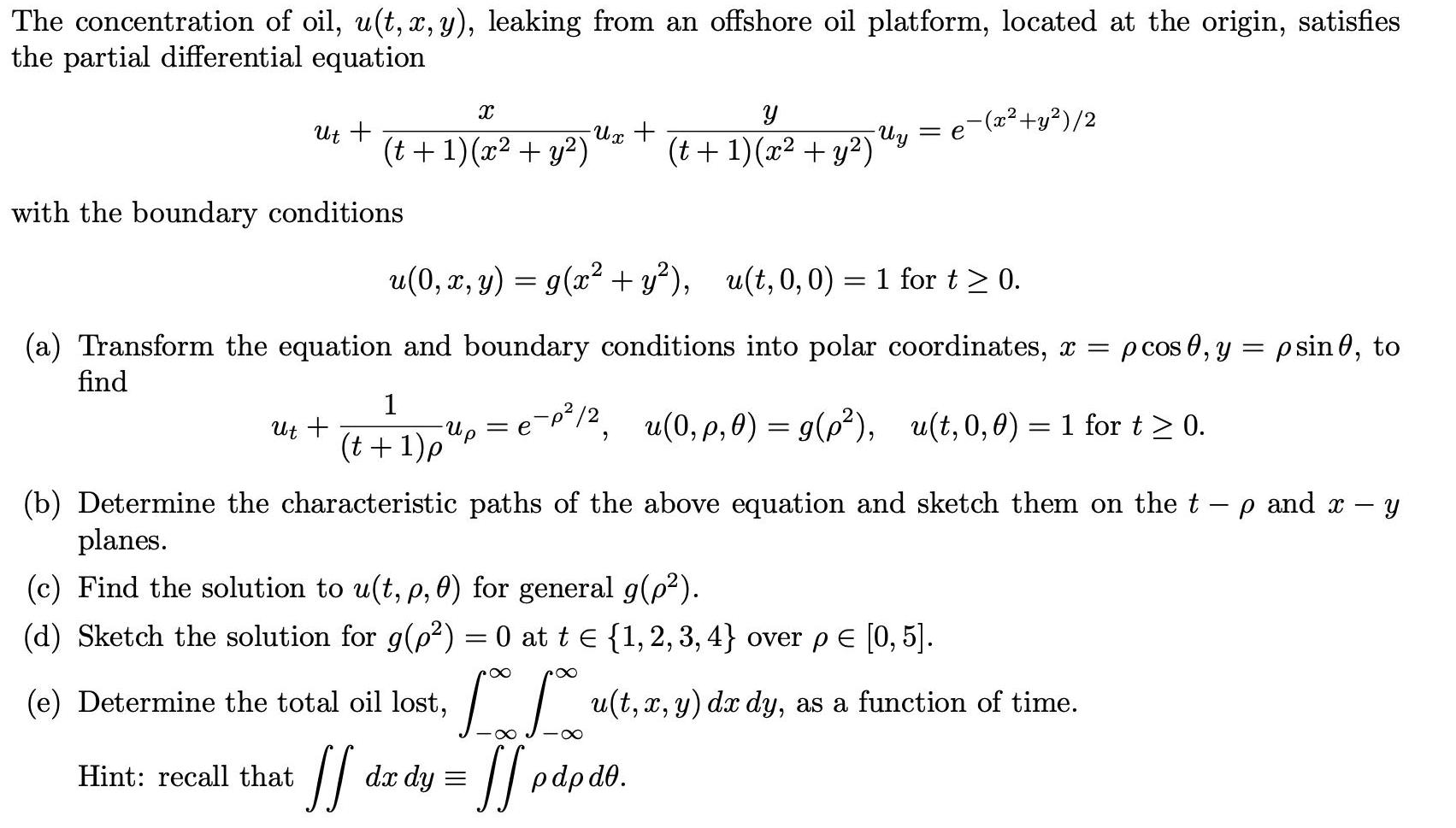
The concentration of oil, u(t, x, y), leaking from an offshore oil platform, located at the origin, satisfies the partial differential equation Ut + -(x+y)/2 = e (t +1)(x2 + y?) (t +1)(x2 + y)Uz+ (t+ 1)(x2 + y2) with the boundary conditions u(0, x, y) = g(x? + y?), u(t, 0,0) = 1 for t > 0. (a) Transform the equation and boundary conditions into polar coordinates, x = find p cos 0, y = psin 0, to Ut + (t +1)p up= e-P*/2, u(0, p,0) = g(p?), u(t, 0,0) = 1 for t > 0. %3D p and x - Y (b) Determine the characteristic paths of the above equation and sketch them on the t planes. (c) Find the solution to u(t, p, 0) for general g(p). (d) Sketch the solution for g(p2) = 0 at t e {1,2, 3, 4} over p e [0, 5]. (e) Determine the total oil lost, | | u(t, x, y) dx dy, as a function of time. dy = || Hint: recall that dx pdp d0.