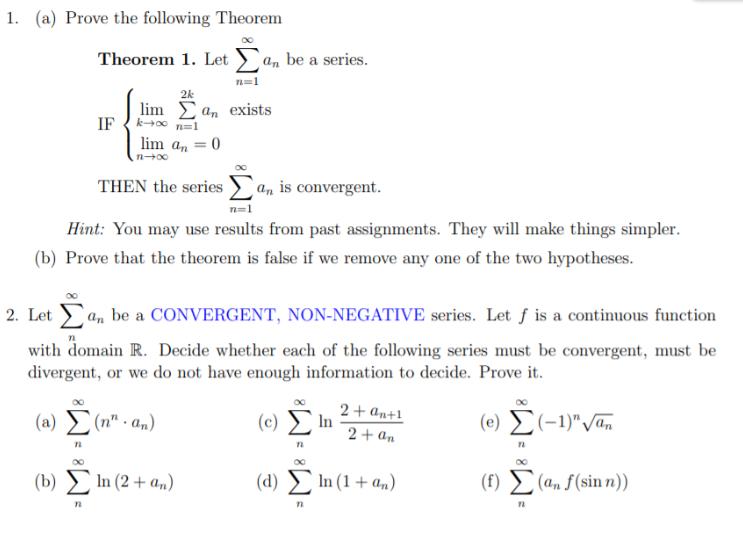
1. (a) Prove the following Theorem 00 Theorem 1. Let an be a series. n=1 2k lim an exists IF kon=1 n lim an 0 84x THEN the seriesa, is convergent. n=1 Hint: You may use results from past assignments. They will make things simpler. (b) Prove that the theorem is false if we remove any one of the two hypotheses. 2. Let an be a CONVERGENT, NON-NEGATIVE series. Let f is a continuous function with domain R. Decide whether each of the following series must be convergent, must be divergent, or we do not have enough information to decide. Prove it. (a) (n".an) (b) In (2+ an) (c) 12 In n 2+an+1 2+ an (d) In (1+an) (e) (-1)" an 72 (f) (an f(sinn)) 12