Acurries under M.I.T

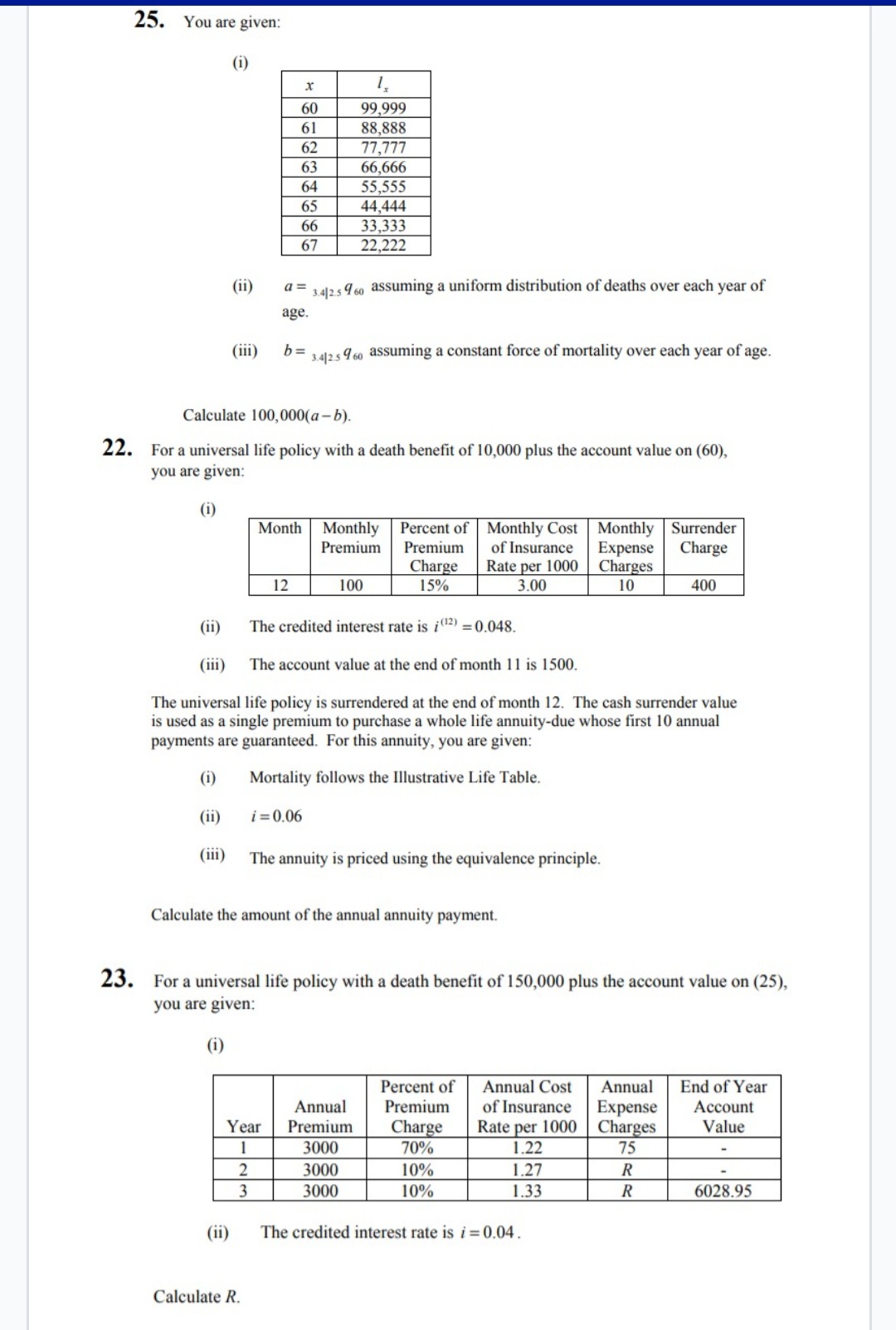
25. You are given: (i) X 60 99,999 61 88,888 62 77,777 63 56,666 64 55,555 65 44,444 66 33,333 67 22,222 (ii) a = 3.42.3 9 60 assuming a uniform distribution of deaths over each year of age. (iii) b= 3.4/25 9 60 assuming a constant force of mortality over each year of age. Calculate 100,000(a -b). 22. For a universal life policy with a death benefit of 10,000 plus the account value on (60), you are given: (i) Month Monthly Percent of Monthly Cost Monthly Surrender Premium Premium of Insurance Expense Charge Charge Rate per 1000 Charges 12 100 15% 3.00 10 400 (1i) The credited interest rate is i () =0.048. (iii) The account value at the end of month 11 is 1500. The universal life policy is surrendered at the end of month 12. The cash surrender value is used as a single premium to purchase a whole life annuity-due whose first 10 annual payments are guaranteed. For this annuity, you are given: (1) Mortality follows the Illustrative Life Table. (ii) i =0.06 (iii) The annuity is priced using the equivalence principle. Calculate the amount of the annual annuity payment. 23. For a universal life policy with a death benefit of 150,000 plus the account value on (25), you are given: (i) Percent of Annual Cost Annual End of Year Annual Premium of Insurance Expense Account Year Premium Charge Rate per 1000 Charges Value 3000 70% 1.22 75 2 3000 10% 1.27 R 3 3000 10% 1.33 R 6028.95 (ii) The credited interest rate is i = 0.04. Calculate R.21. You are pricing an automobile insurance on (x). The insurance pays 10,000 immediately if (x) gets into an accident within 5 years of issue. The policy pays only for the first accident and has no other benefits. You are given: (i) You model (x)'s driving status as a multi-state model with the following 3 states: 0 - low risk, without an accident 1 - high risk, without an accident 2 - has had an accident (ii) (x) is initially in state 0. (iii) The following transition intensities for 0St $5: W xHI = 0.20+0.10r THI = 0.05+0.051 12 =0.15+0.0172 No other transitions are possible. (iv) 3 PT =0.4174 (v) 6=0.02 (vi) The continuous function g(t) is such that the expected present value of the benefit up to time a equals [" g(1)di, OSas5, where is the time of the first accident. Calculate g(3)