Answered step by step
Verified Expert Solution
Question
1 Approved Answer
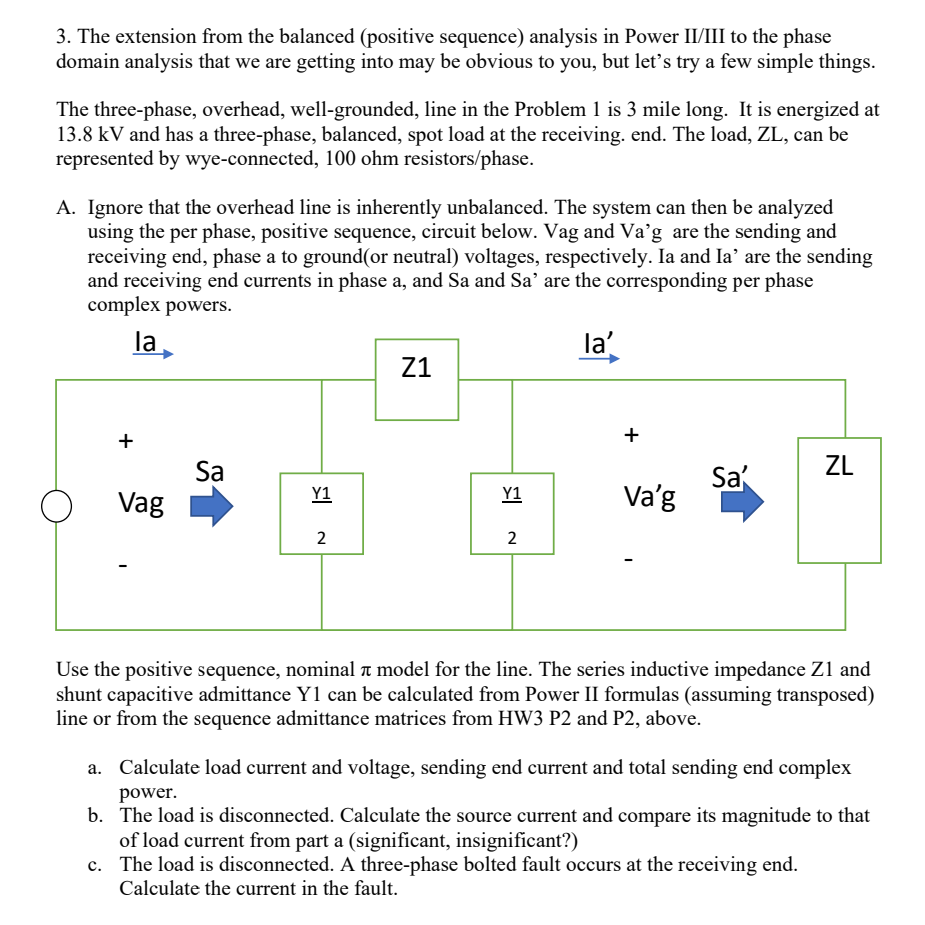
all informatin should be included, if not then the proffesor said to state the assumptions 3. The extension from the balanced (positive sequence) analysis in
all informatin should be included, if not then the proffesor said to state the assumptions
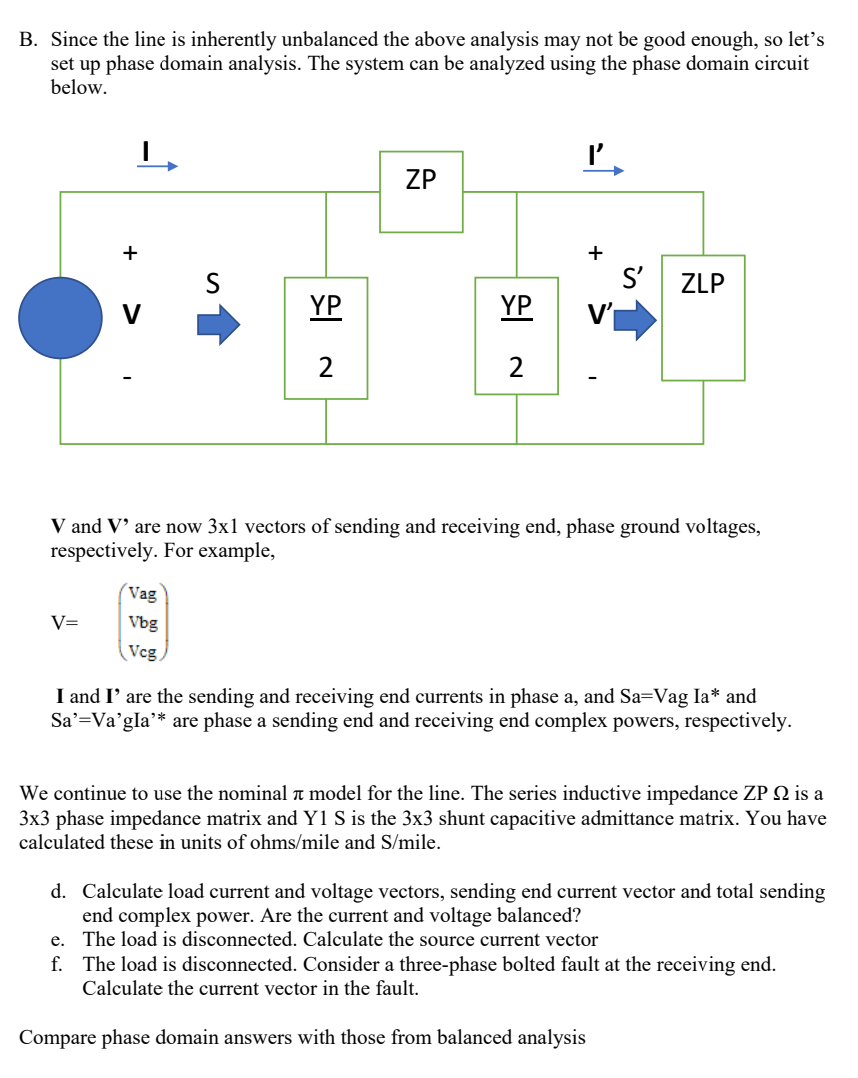
3. The extension from the balanced (positive sequence) analysis in Power II/III to the phase domain analysis that we are getting into may be obvious to you, but let's try a few simple things. The three-phase, overhead, well-grounded, line in the Problem 1 is 3 mile long. It is energized at 13.8 kV and has a three-phase, balanced, spot load at the receiving. end. The load, ZL, can be represented by wye-connected, 100 ohm resistors/phase. A. Ignore that the overhead line is inherently unbalanced. The system can then be analyzed using the per phase, positive sequence, circuit below. Vag and Vag are the sending and receiving end, phase a to ground(or neutral) voltages, respectively. Ia and la are the sending and receiving end currents in phase a, and Sa and Sa are the corresponding per phase complex powers. la la' Z1 + + Sa ZL Sa' Vag Y1 Y1 Va'g 2 2 Use the positive sequence, nominal a model for the line. The series inductive impedance Zl and shunt capacitive admittance Y1 can be calculated from Power II formulas (assuming transposed) line or from the sequence admittance matrices from HW3 P2 and P2, above. a. Calculate load current and voltage, sending end current and total sending end complex power. b. The load is disconnected. Calculate the source current and compare its magnitude to that of load current from part a (significant, insignificant?) c. The load is disconnected. A three-phase bolted fault occurs at the receiving end. Calculate the current in the fault. B. Since the line is inherently unbalanced the above analysis may not be good enough, so let's set up phase domain analysis. The system can be analyzed using the phase domain circuit below. ZP + + S ZLP YP S V' YP 2 2 V and V' are now 3x1 vectors of sending and receiving end, phase ground voltages, respectively. For example, Vag Vbg V= I and I' are the sending and receiving end currents in phase a, and Sa=Vag Ia* and Sa=Vagla'* are phase a sending end and receiving end complex powers, respectively. We continue to use the nominal a model for the line. The series inductive impedance ZP 2 is a 3x3 phase impedance matrix and Y1 S is the 3x3 shunt capacitive admittance matrix. You have calculated these in units of ohms/mile and S/mile. d. Calculate load current and voltage vectors, sending end current vector and total sending end complex power. Are the current and voltage balanced? e. The load is disconnected. Calculate the source current vector f. The load is disconnected. Consider a three-phase bolted fault at the receiving end. Calculate the current vector in the fault. Compare phase domain answers with those from balanced analysis 3. The extension from the balanced (positive sequence) analysis in Power II/III to the phase domain analysis that we are getting into may be obvious to you, but let's try a few simple things. The three-phase, overhead, well-grounded, line in the Problem 1 is 3 mile long. It is energized at 13.8 kV and has a three-phase, balanced, spot load at the receiving. end. The load, ZL, can be represented by wye-connected, 100 ohm resistors/phase. A. Ignore that the overhead line is inherently unbalanced. The system can then be analyzed using the per phase, positive sequence, circuit below. Vag and Vag are the sending and receiving end, phase a to ground(or neutral) voltages, respectively. Ia and la are the sending and receiving end currents in phase a, and Sa and Sa are the corresponding per phase complex powers. la la' Z1 + + Sa ZL Sa' Vag Y1 Y1 Va'g 2 2 Use the positive sequence, nominal a model for the line. The series inductive impedance Zl and shunt capacitive admittance Y1 can be calculated from Power II formulas (assuming transposed) line or from the sequence admittance matrices from HW3 P2 and P2, above. a. Calculate load current and voltage, sending end current and total sending end complex power. b. The load is disconnected. Calculate the source current and compare its magnitude to that of load current from part a (significant, insignificant?) c. The load is disconnected. A three-phase bolted fault occurs at the receiving end. Calculate the current in the fault. B. Since the line is inherently unbalanced the above analysis may not be good enough, so let's set up phase domain analysis. The system can be analyzed using the phase domain circuit below. ZP + + S ZLP YP S V' YP 2 2 V and V' are now 3x1 vectors of sending and receiving end, phase ground voltages, respectively. For example, Vag Vbg V= I and I' are the sending and receiving end currents in phase a, and Sa=Vag Ia* and Sa=Vagla'* are phase a sending end and receiving end complex powers, respectively. We continue to use the nominal a model for the line. The series inductive impedance ZP 2 is a 3x3 phase impedance matrix and Y1 S is the 3x3 shunt capacitive admittance matrix. You have calculated these in units of ohms/mile and S/mile. d. Calculate load current and voltage vectors, sending end current vector and total sending end complex power. Are the current and voltage balanced? e. The load is disconnected. Calculate the source current vector f. The load is disconnected. Consider a three-phase bolted fault at the receiving end. Calculate the current vector in the fault. Compare phase domain answers with those from balanced analysisStep by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


