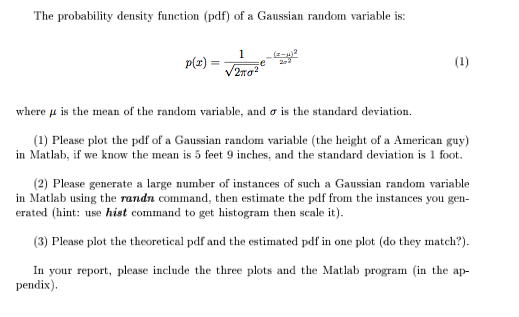
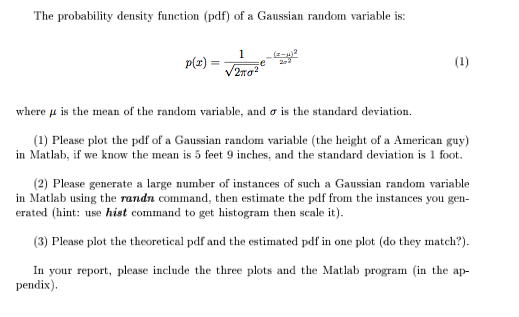
Question
Allow X to signify the voltage at the yield of a mouthpiece, and assume that X has a uniform circulation on the stretch from ?1


Allow X to signify the voltage at the yield of a mouthpiece, and assume that X has a uniform circulation on the stretch from ?1 to 1. The voltage is handled by a "hard limiter" with cutoff esteems ?0.5 and 0.5, so the limiter yield is an arbitrary variable Y identified with X by Y = X if |X| ? 0.5, Y = 0.5 if X > 0.5, and Y = ?0.5 if X
(a) What is P(Y = 0.5)?
(b) Obtain the combined appropriation capacity of Y.
If it's not too much trouble, show your work, much obliged!
A firm uses pattern projection and occasional components to reproduce deals for a given time frame period. It doles out "0" if deals fall, "1" if deals are consistent, "2" if deals rise reasonably, and "3" if deals rise a ton. The test system creates the accompanying yield.
0 1 0 2 0 1 0 3 2 0 2 1 2 3 1 2 0 2 0 3 0 2 1 0 1
Gauge the likelihood that deals will rise tolerably.
a
0.258
b
0.233
c
0.312
@2@
d
0.226
Consider the examination of flipping a reasonable coin until two heads or two tails show up in
progression.
(a) Describe the example space.
(b) What is the likelihood that the analysis closes before the 6th throw?
(c) What is the likelihood that the investigation closes after a much number of throws?
(d) Given that the examination closes with two heads, what is the likelihood that the
analyze closes before the 6th throw?
(e) Given that the investigation doesn't end before the third throw, what is the likelihood that
the analysis doesn't end after the 6th throw?
Allow X to mean the measure of time a book on two-hour hold is really looked at, and assume the cdf is the accompanying. F(x) = 0 if x = 5. Utilize the cdf to acquire the accompanying. (On the off chance that vital, round your response to four decimal spots.)
(g) Calculate V(X) and ?x.
(h) If the borrower is charged a sum h(X) = X2 when checkout length is X, figure the normal charge E[h(X)].



Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


