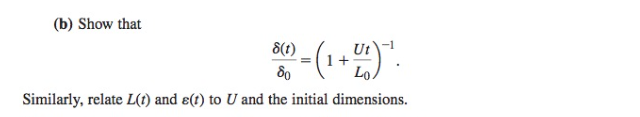
Also part of problem 2-6 as reference
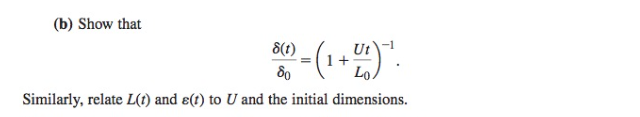
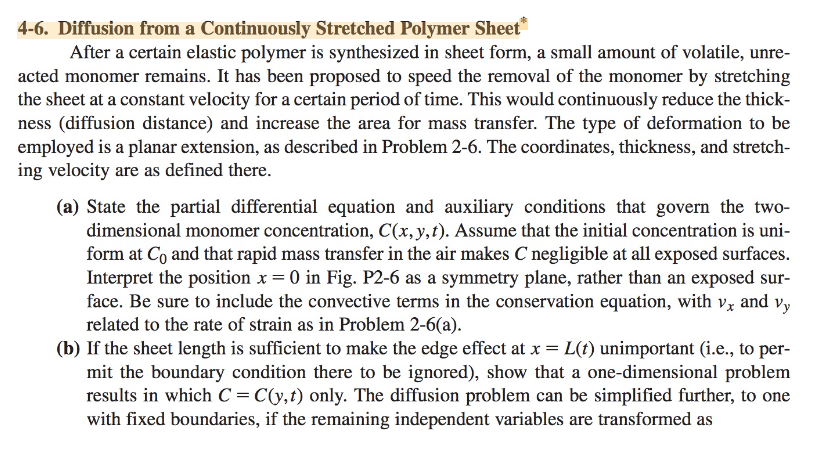
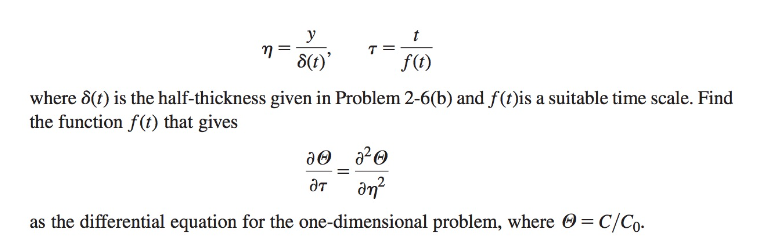
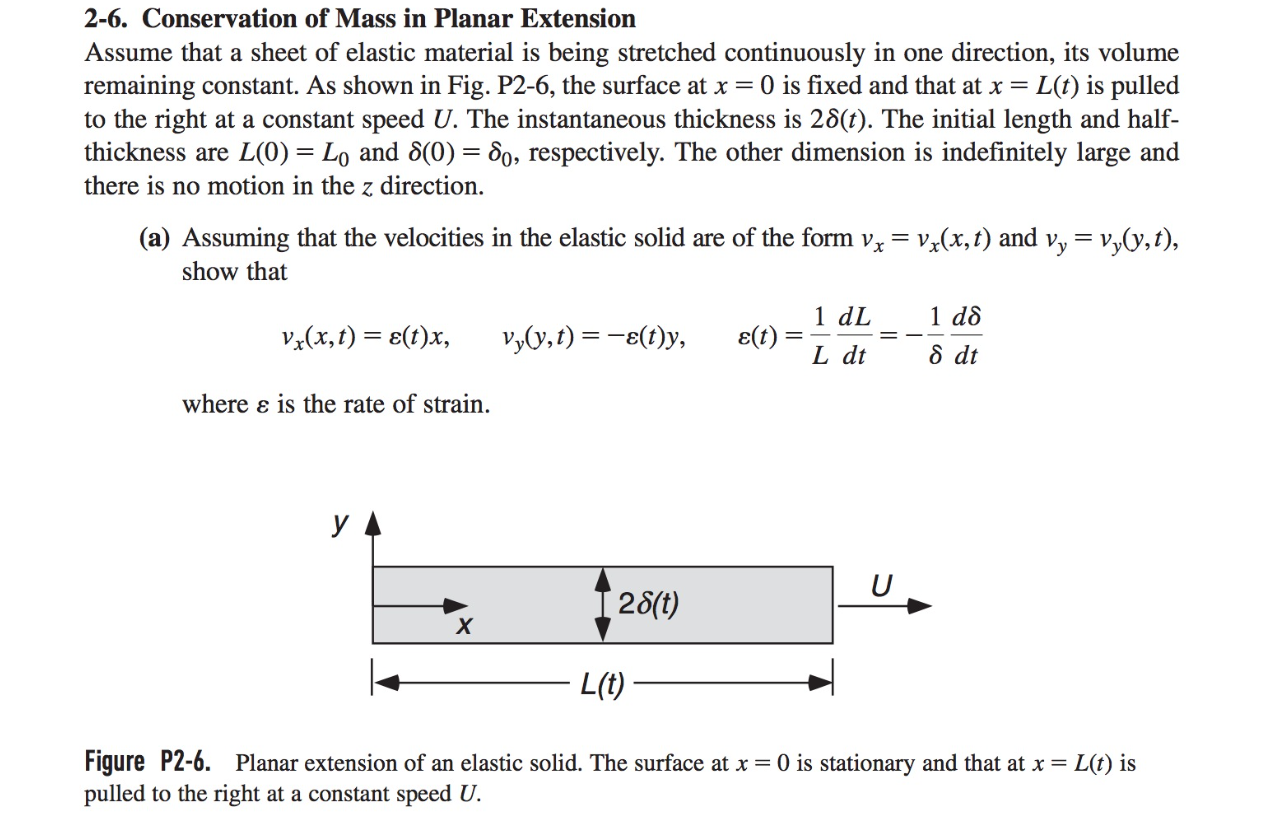
4-6. Diffusion from a Continuously Stretched Polymer Sheet After a certain elastic polymer is synthesized in sheet form, a small amount of volatile, unreacted monomer remains. It has been proposed to speed the removal of the monomer by stretching the sheet at a constant velocity for a certain period of time. This would continuously reduce the thickness (diffusion distance) and increase the area for mass transfer. The type of deformation to be employed is a planar extension, as described in Problem 2-6. The coordinates, thickness, and stretching velocity are as defined there. (a) State the partial differential equation and auxiliary conditions that govern the twodimensional monomer concentration, C(x,y,t). Assume that the initial concentration is uniform at C0 and that rapid mass transfer in the air makes C negligible at all exposed surfaces. Interpret the position x=0 in Fig. P2-6 as a symmetry plane, rather than an exposed surface. Be sure to include the convective terms in the conservation equation, with vx and vy related to the rate of strain as in Problem 2-6(a). (b) If the sheet length is sufficient to make the edge effect at x=L(t) unimportant (i.e., to permit the boundary condition there to be ignored), show that a one-dimensional problem results in which C=C(y,t) only. The diffusion problem can be simplified further, to one with fixed boundaries, if the remaining independent variables are transformed as =(t)y,=f(t)t where (t) is the half-thickness given in Problem 2-6(b) and f(t) is a suitable time scale. Find the function f(t) that gives =22 as the differential equation for the one-dimensional problem, where =C/C0. 2-6. Conservation of Mass in Planar Extension Assume that a sheet of elastic material is being stretched continuously in one direction, its volume remaining constant. As shown in Fig. P2-6, the surface at x=0 is fixed and that at x=L(t) is pulled to the right at a constant speed U. The instantaneous thickness is 2(t). The initial length and halfthickness are L(0)=L0 and (0)=0, respectively. The other dimension is indefinitely large and there is no motion in the z direction. (a) Assuming that the velocities in the elastic solid are of the form vx=vx(x,t) and vy=vy(y,t), show that vx(x,t)=(t)x,vy(y,t)=(t)y,(t)=L1dtdL=1dtd where is the rate of strain. Figure P2-6. Planar extension of an elastic solid. The surface at x=0 is stationary and that at x=L(t) is pulled to the right at a constant speed U. (b) Show that 0(t)=(1+L0Ut)1. Similarly, relate L(t) and (t) to U and the initial dimensions. 4-6. Diffusion from a Continuously Stretched Polymer Sheet After a certain elastic polymer is synthesized in sheet form, a small amount of volatile, unreacted monomer remains. It has been proposed to speed the removal of the monomer by stretching the sheet at a constant velocity for a certain period of time. This would continuously reduce the thickness (diffusion distance) and increase the area for mass transfer. The type of deformation to be employed is a planar extension, as described in Problem 2-6. The coordinates, thickness, and stretching velocity are as defined there. (a) State the partial differential equation and auxiliary conditions that govern the twodimensional monomer concentration, C(x,y,t). Assume that the initial concentration is uniform at C0 and that rapid mass transfer in the air makes C negligible at all exposed surfaces. Interpret the position x=0 in Fig. P2-6 as a symmetry plane, rather than an exposed surface. Be sure to include the convective terms in the conservation equation, with vx and vy related to the rate of strain as in Problem 2-6(a). (b) If the sheet length is sufficient to make the edge effect at x=L(t) unimportant (i.e., to permit the boundary condition there to be ignored), show that a one-dimensional problem results in which C=C(y,t) only. The diffusion problem can be simplified further, to one with fixed boundaries, if the remaining independent variables are transformed as =(t)y,=f(t)t where (t) is the half-thickness given in Problem 2-6(b) and f(t) is a suitable time scale. Find the function f(t) that gives =22 as the differential equation for the one-dimensional problem, where =C/C0. 2-6. Conservation of Mass in Planar Extension Assume that a sheet of elastic material is being stretched continuously in one direction, its volume remaining constant. As shown in Fig. P2-6, the surface at x=0 is fixed and that at x=L(t) is pulled to the right at a constant speed U. The instantaneous thickness is 2(t). The initial length and halfthickness are L(0)=L0 and (0)=0, respectively. The other dimension is indefinitely large and there is no motion in the z direction. (a) Assuming that the velocities in the elastic solid are of the form vx=vx(x,t) and vy=vy(y,t), show that vx(x,t)=(t)x,vy(y,t)=(t)y,(t)=L1dtdL=1dtd where is the rate of strain. Figure P2-6. Planar extension of an elastic solid. The surface at x=0 is stationary and that at x=L(t) is pulled to the right at a constant speed U. (b) Show that 0(t)=(1+L0Ut)1. Similarly, relate L(t) and (t) to U and the initial dimensions