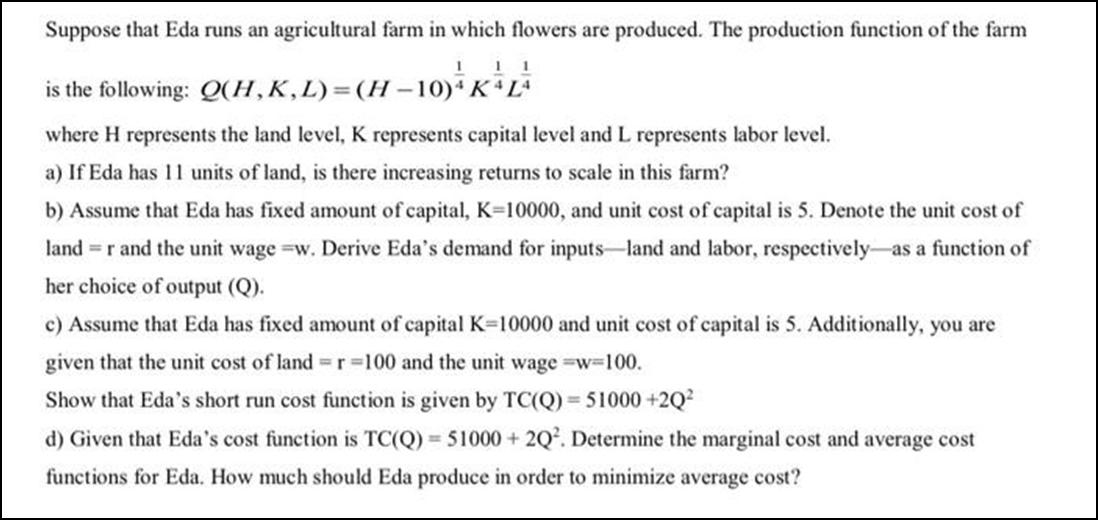
Suppose that Eda runs an agricultural farm in which flowers are produced. The production function of the farm 111 is the following: Q(H, K, L)=(H-10)+ KALA where H represents the land level, K represents capital level and L represents labor level. a) If Eda has 11 units of land, is there increasing returns to scale in this farm? b) Assume that Eda has fixed amount of capital, K=10000, and unit cost of capital is 5. Denote the unit cost of land = r and the unit wage=w. Derive Eda's demand for inputs-land and labor, respectively as a function of her choice of output (Q). c) Assume that Eda has fixed amount of capital K=10000 and unit cost of capital is 5. Additionally, you are given that the unit cost of land =r=100 and the unit wage=w=100. Show that Eda's short run cost function is given by TC(Q) = 51000 +2Q d) Given that Eda's cost function is TC(Q) = 51000 + 2Q. Determine the marginal cost and average cost functions for Eda. How much should Eda produce in order to minimize average cost?